(Solved): Determine if the two functions are inverse to each other: \( f(x)=4 x-8 \) and \( g(x)=\frac{x}{4} ...

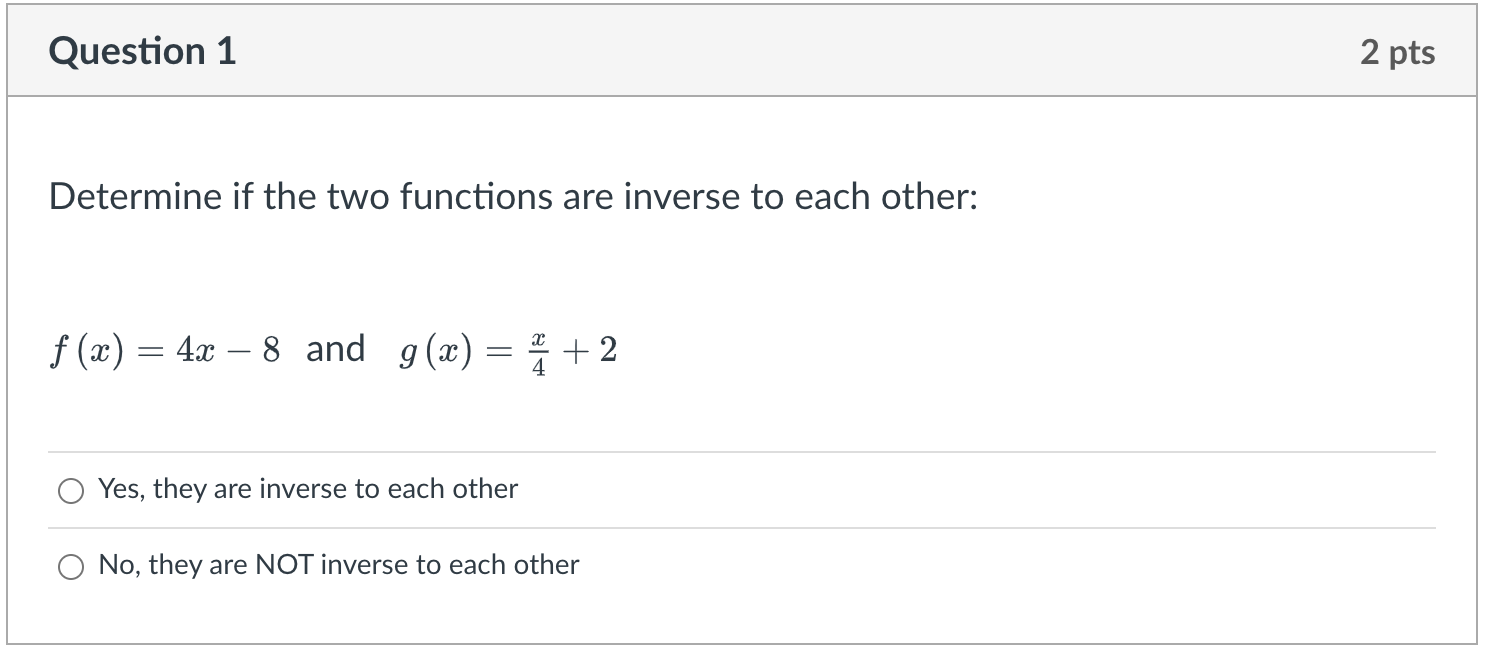
Determine if the two functions are inverse to each other: \( f(x)=4 x-8 \) and \( g(x)=\frac{x}{4}+2 \) Yes, they are inverse to each other No, they are NOT inverse to each other Determine if the two functions are inverse to each other: \( f(x)=x^{3}-8 \) and \( g(x)=\sqrt[3]{x+8} \) Yes, they are inverse to each other No, they are NOT inverse to each other Determine the inverse of the function \[ f(x)=\frac{2}{3+x} \] \[ \begin{array}{l} f^{-1}(x)=\frac{3+x}{2} \\ f^{-1}(x)=\frac{3+x}{2+x} \\ f^{-1}(x)=\frac{2}{x}+3 \\ f^{-1}(x)=\frac{2}{x}-3 \end{array} \] None Determine the inverse of the function \[ f(x)=\frac{2 x-3}{4+x} \] \[ \begin{array}{l} f^{-1}(x)=\frac{4 x+3}{2-x} \\ f^{-1}(x)=\frac{4 x-3}{2-x} \\ f^{-1}(x)=\frac{4 x+3}{2+x} \\ f^{-1}(x)=\frac{4 x-3}{2+x} \end{array} \] None Determine the inverse of the function \[ f(x)=x^{\frac{2}{3}}-4 \] \[ \begin{array}{l} f^{-1}(x)=(4+x)^{\frac{3}{2}} \\ f^{-1}(x)=(4-x)^{\frac{3}{2}} \\ f^{-1}(x)=4+x^{\frac{3}{2}} \\ f^{-1}(x)=(4+x)^{\frac{2}{3}} \end{array} \] None