(Solved): Describe how Maxwell equations in vector(right part) form can be developed from the corresponding in ...

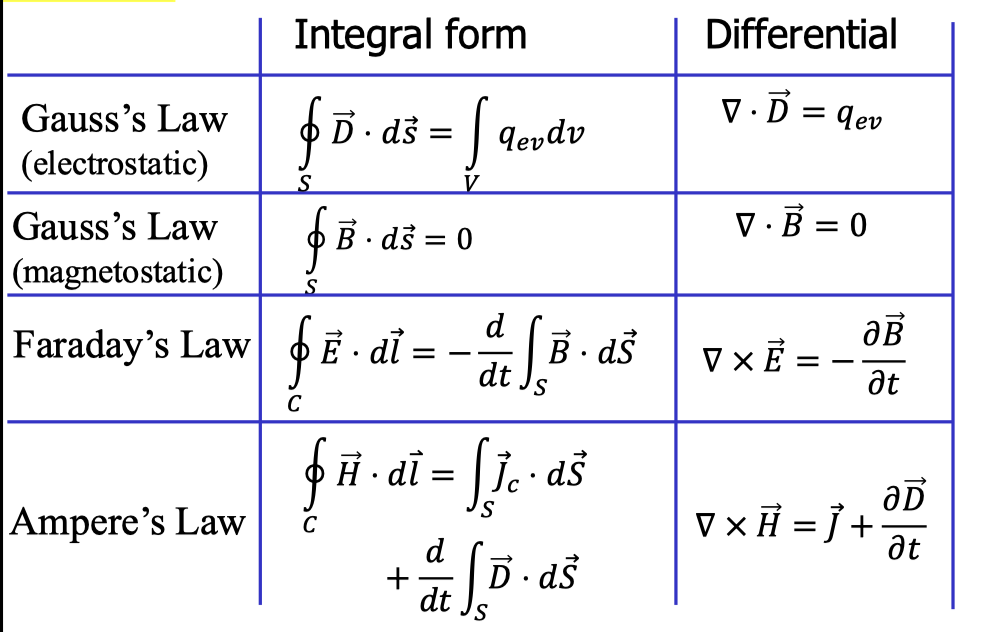
Describe how Maxwell equations in vector(right part) form can be developed from the corresponding integral(right part) form.

I saw others answer from here, they are all wrong. ALL WRONG!

So, could you write all details for how to doing the question?

Thanks so much.

Thanks so much. 

\begin{tabular}{l|l|c|} & Integral form & Differential \\ \hline Gauss's Law (electrostatic) & \( \oint_{S} \vec{D} \cdot d \vec{s}=\int_{V} q_{e v} d v \) & \( \nabla \cdot \vec{D}=q_{e v} \) \\ \hline Gauss's Law (magnetostatic) & \( \oint_{S} \vec{B} \cdot d \vec{s}=0 \) & \( \nabla \cdot \vec{B}=0 \) \\ \hline Faraday's Law & \( \oint_{C} \vec{E} \cdot d \vec{l}=-\frac{d}{d t} \int_{S} \vec{B} \cdot d \vec{S} \) & \( \nabla \times \vec{E}=-\frac{\partial \vec{B}}{\partial t} \) \\ \hline Ampere's Law & \( \oint_{C} \vec{H} \cdot d \vec{l}=\int_{S} \overrightarrow{J_{c}} \cdot d \vec{S} \) & \\ & \( +\frac{d}{d t} \int_{S} \vec{D} \cdot d \vec{S} \) & \( \nabla \times \vec{H}=\vec{J}+\frac{\partial \vec{D}}{\partial t} \) \end{tabular}