(Solved): Derive expressions for the vector Laplacian, \( \nabla^{2} \mathbf{V} \) in cylindrical coordinate ...

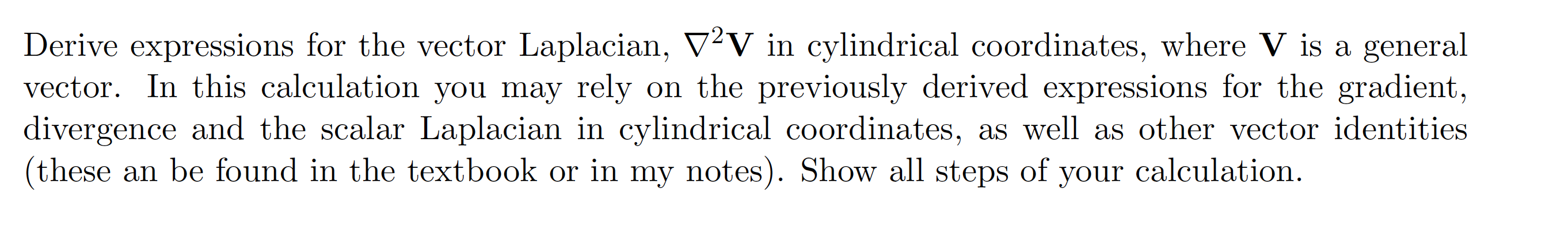
Derive expressions for the vector Laplacian, \( \nabla^{2} \mathbf{V} \) in cylindrical coordinates, where \( \mathbf{V} \) is a general vector. In this calculation you may rely on the previously derived expressions for the gradient, divergence and the scalar Laplacian in cylindrical coordinates, as well as other vector identities (these an be found in the textbook or in my notes). Show all steps of your calculation.