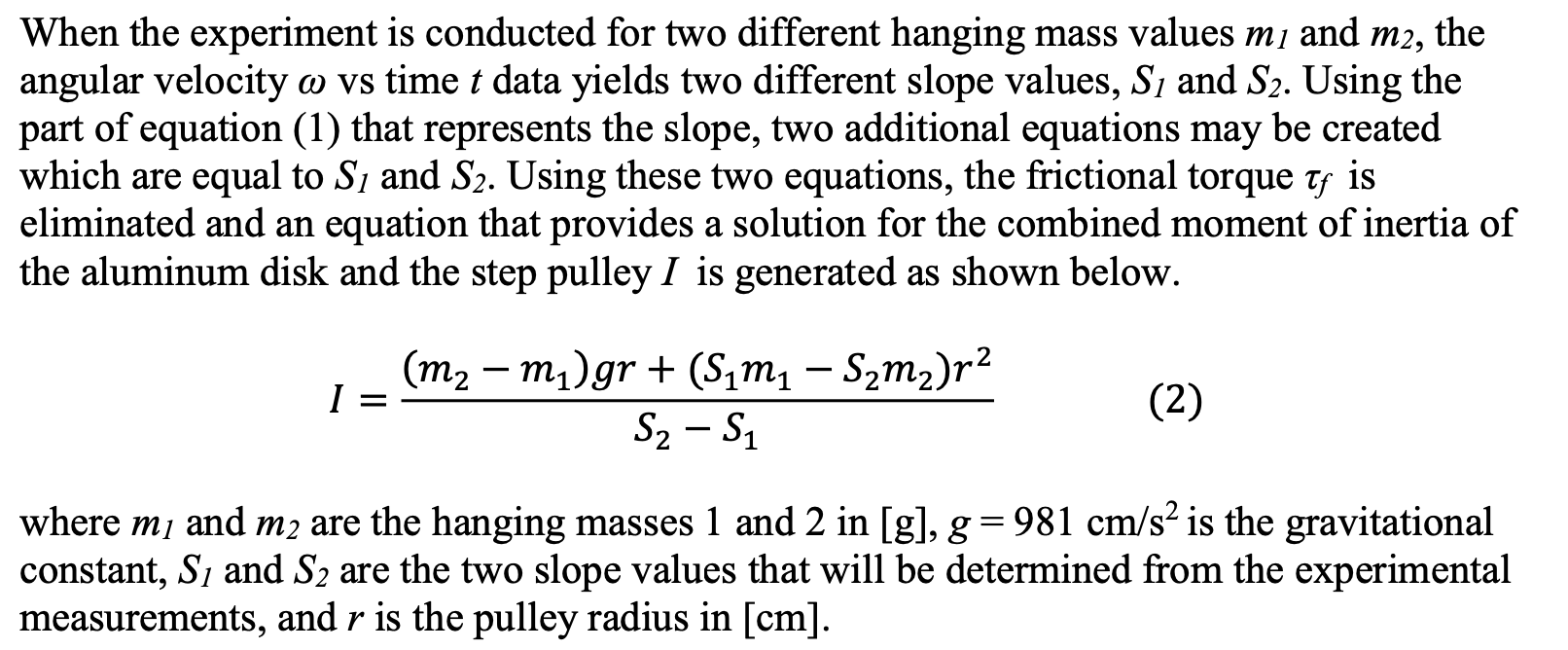(Solved): - Derive equation (2) using the hints given in the theory section of this handout.     ...

- Derive equation (2) using the hints given in the theory section of this handout.

 

 

 

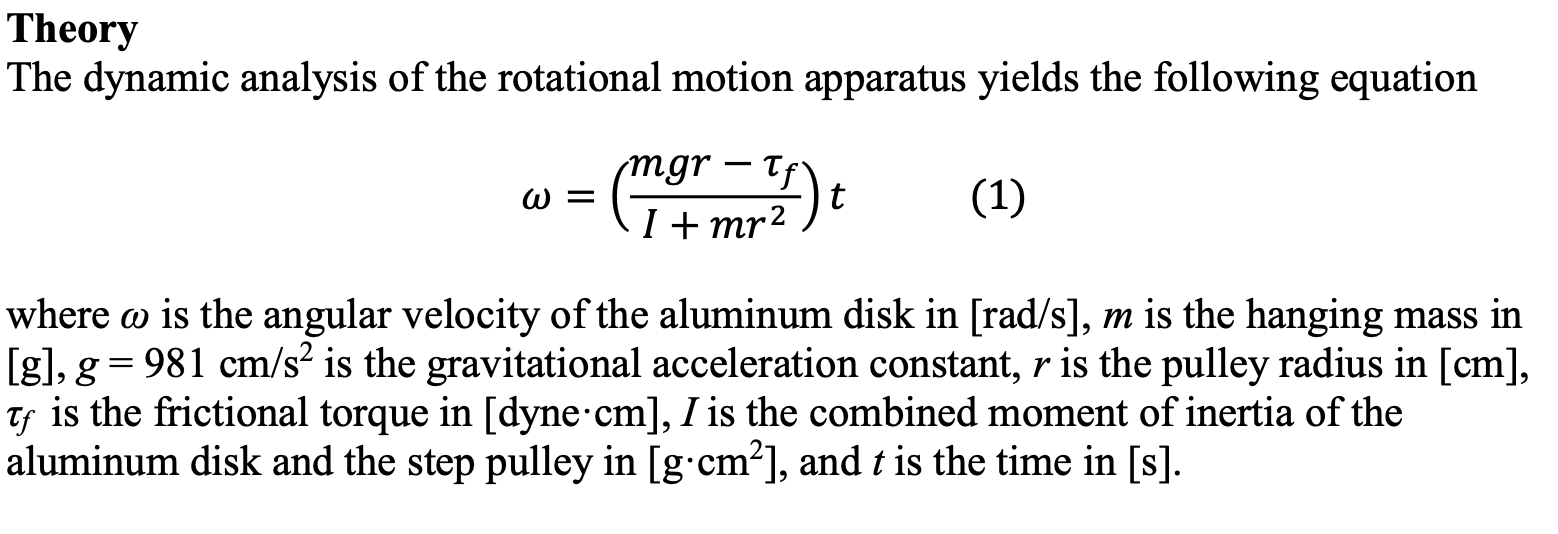
Theory The dynamic analysis of the rotational motion apparatus yields the following equation W= mgr - Tf (mgr (1) I- t (1) where w is the angular velocity of the aluminum disk in [rad/s], m is the hanging mass in [g], g = 981 cm/s² is the gravitational acceleration constant, r is the pulley radius in [cm], Tf is the frictional torque in [dyne cm], I is the combined moment of inertia of the aluminum disk and the step pulley in [g-cm²], and t is the time in [s]. When the experiment is conducted for two different hanging mass values mi and m2, the angular velocity @ vs time t data yields two different slope values, S? and S?. Using the part of equation (1) that represents the slope, two additional equations may be created which are equal to S? and S?. Using these two equations, the frictional torque tf is eliminated and an equation that provides a solution for the combined moment of inertia of the aluminum disk and the step pulley I is generated as shown below. Tf I = (m? ? m?)gr + (S?m? ? S?m?)r² S? - S? (2) where my and m2 are the hanging masses 1 and 2 in [g], g = 981 cm/s² is the gravitational constant, S, and S2 are the two slope values that will be determined from the experimental measurements, and r is the pulley radius in [cm].