(Solved): Cylindrical (Polar) Coordinates (16 points) (a) Consider a point P at (x,y,z)=(4,3,0)m. Draw a dia ...

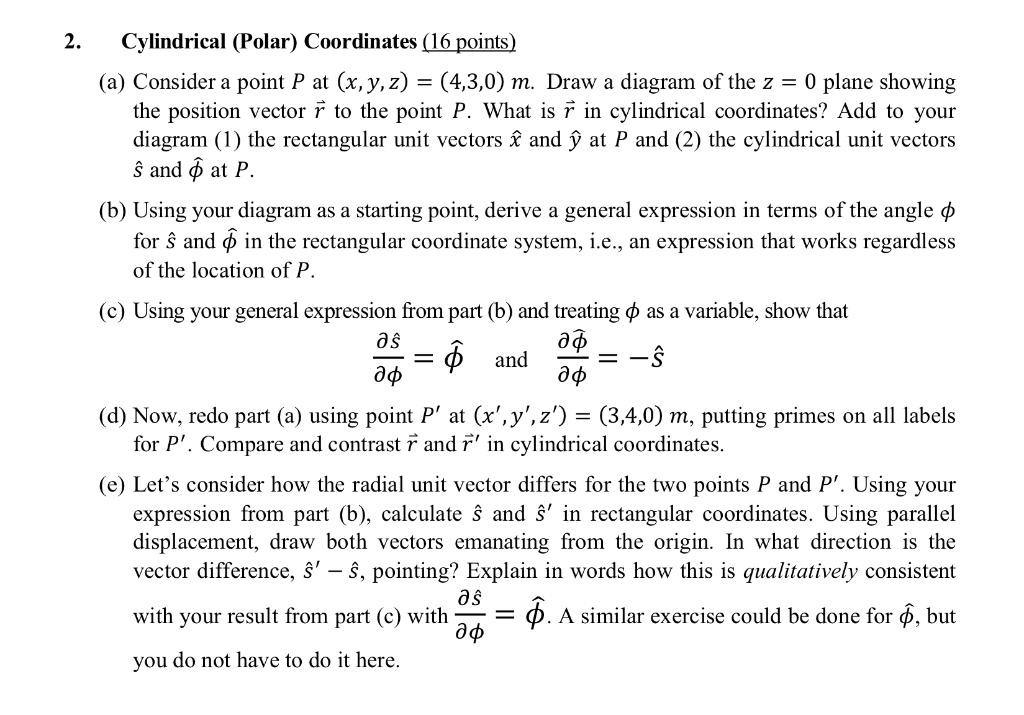
Cylindrical (Polar) Coordinates (16 points) (a) Consider a point $$P$$ at $$(x,y,z)=(4,3,0)m$$. Draw a diagram of the $$z=0$$ plane showing the position vector $$\vec{r}$$ to the point $$P$$. What is $$\vec{r}$$ in cylindrical coordinates? Add to your diagram (1) the rectangular unit vectors $$\hat{x}$$ and $$\hat{y}$$ at $$P$$ and (2) the cylindrical unit vectors $$\hat{s}$$ and $$\widehat{?}$$ at $$P$$. (b) Using your diagram as a starting point, derive a general expression in terms of the angle $$?$$ for $$\hat{S}$$ and $$\widehat{?}$$ in the rectangular coordinate system, i.e., an expression that works regardless of the location of $$P$$. (c) Using your general expression from part (b) and treating $$?$$ as a variable, show that $$\frac{?\hat{s}}{??}=\widehat{?}\text{ and }\frac{?\widehat{?}}{??}=?\hat{S}$$ (d) Now, redo part (a) using point $$P^{?}$$ at $$\left(x^{?},y^{?},z^{?}\right)=(3,4,0)m$$, putting primes on all labels for $$P^{?}$$. Compare and contrast $$\vec{r}$$ and $${\vec{r}}^{?}$$ in cylindrical coordinates. (e) Let's consider how the radial unit vector differs for the two points $$P$$ and $$P^{?}$$. Using your expression from part (b), calculate $$\hat{s}$$ and $${\hat{s}}^{?}$$ in rectangular coordinates. Using parallel displacement, draw both vectors emanating from the origin. In what direction is the vector difference, $${\hat{s}}^{?}?\hat{s}$$, pointing? Explain in words how this is qualitatively consistent with your result from part (c) with $$\frac{?\hat{s}}{??}=\widehat{?}$$. A similar exercise could be done for $$\widehat{?}$$, but you do not have to do it here.