(Solved): Cylindrical Can 1 Find the maximum volume of a cylindrical can that can be made from \( 680 \mathr ...

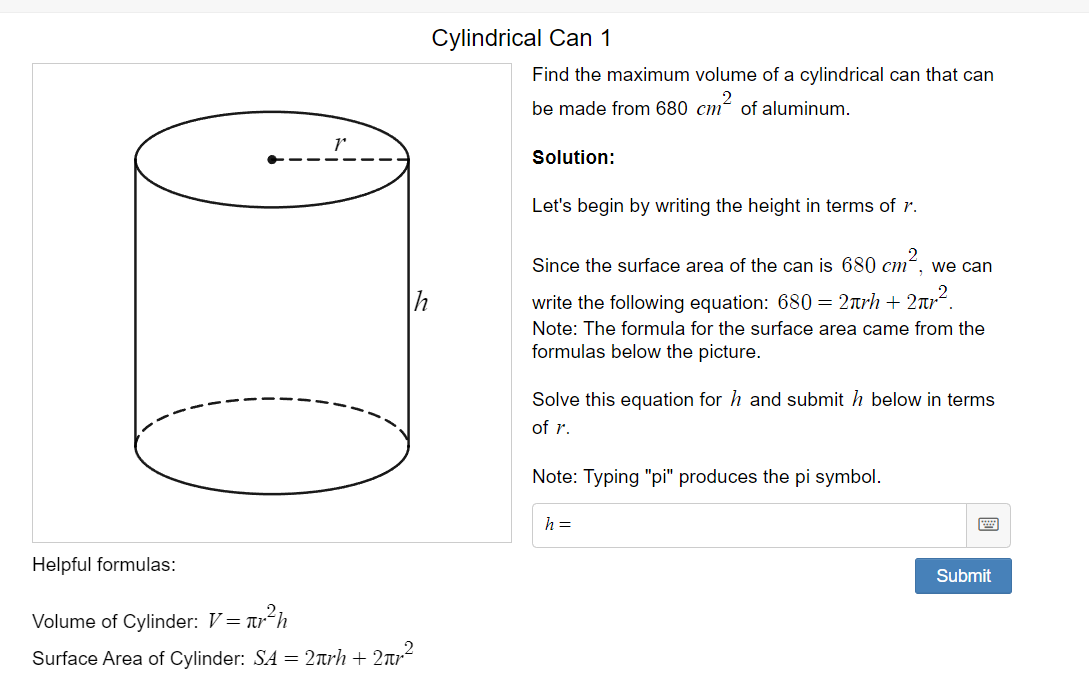
Cylindrical Can 1 Find the maximum volume of a cylindrical can that can be made from \( 680 \mathrm{~cm}^{2} \) of aluminum. Solution: Let's begin by writing the height in terms of \( r \). Since the surface area of the can is \( 680 \mathrm{~cm}^{2} \), we can write the following equation: \( 680=2 \pi r h+2 \pi r^{2} \). Note: The formula for the surface area came from the formulas below the picture. Solve this equation for \( h \) and submit \( h \) below in terms of \( r \). Note: Typing "pi" produces the pi symbol. 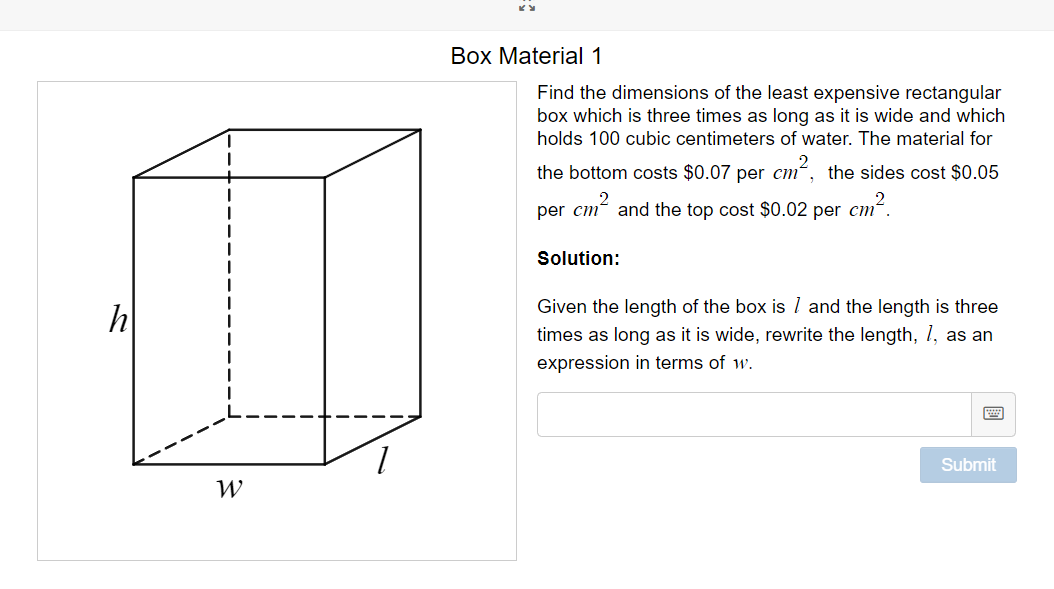Helpful formulas: Volume of Cylinder: \( V=\pi r^{2} h \) Surface Area of Cylinder: \( S A=2 \pi r h+2 \pi r^{2} \) Find the dimensions of the least expensive rectangular box which is three times as long as it is wide and which holds 100 cubic centimeters of water. The material for the bottom costs \( \$ 0.07 \) per \( \mathrm{cm}^{2} \), the sides cost \( \$ 0.05 \) per \( \mathrm{cm}^{2} \) and the top cost \( \$ 0.02 \) per \( \mathrm{cm}^{2} \). Solution: Given the length of the box is \( l \) and the length is three times as long as it is wide, rewrite the length, \( l \), as an expression in terms of \( w \).