Home /
Expert Answers /
Physics /
cpcv-t-tp-v-tv-p-a-show-that-for-the-ideal-gas-this-yields-the-we-pa284
(Solved): CPCV=T(TP)V(TV)P a) Show that for the ideal gas this yields the we ...
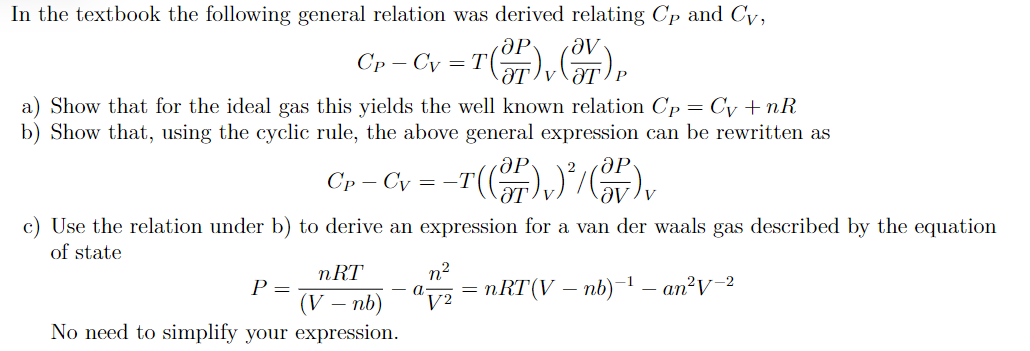
a) Show that for the ideal gas this yields the well known relation b) Show that, using the cyclic rule, the above general expression can be rewritten as c) Use the relation under b) to derive an expression for a van der waals gas described b of state No need to simplify your expression.
Expert Answer
To show that for an ideal gas, the relation Cp = Cv + nR holds using the given equation , we can apply the ideal gas law and the definition of specific heat capacities.Starting with the given equation: We know that for an ideal gas, the specific heat capacities are defined as follows: (heat capacity at constant pressure) (heat capacity at constant volume)Using the first law of thermodynamics, we have: (change in heat energy = change in internal energy + work done)For an ideal gas, the change in internal energy (dU) only depends on the change in temperature (dT). Therefore, we can write: Substituting this into the equation for heat capacity at constant pressure (Cp), we have: Cp = (?Q/?T)p = dU/dT + P(dV/dT)pNow, let's consider the ideal gas law: PV = nRTTaking the partial derivative of this equation with respect to time (t) at constant volume (V), we get: V(?P/?t)v = nRSubstituting this into the expression for Cp, we have: Cp = dU/dT + P(dV/dT)p = Cv + (nR/V)(dV/dT)pSince nR/V = P (using the ideal gas law), we have: Cp = Cv + P(dV/dT)pTherefore, for an ideal gas, the relation Cp = Cv + nR holds true.Now, to express the relation in terms of the number of moles (n) and the ideal gas constant (R), we can use the ideal gas law: PV = nRTSolving for P, we have: P = nRT/VSubstituting this back into the equation for Cp, we get: Cp = Cv + P(dV/dT)p = Cv + (nRT/V)(dV/dT)pSince the partial derivative (?V/?T)p represents the change in volume with respect to temperature at constant pressure, it is related to the thermal expansion coefficient (?) of the gas: (?V/?T)p = V?Therefore, we have: Cp = Cv + P(dV/dT)p = Cv + P(V?) = Cv + nRT?/V = Cv + nR?So, the relation for an ideal gas can be expressed as: Cp = Cv + nRThus, we have shown that for an ideal gas, Cp = Cv + nR, which is the well-known relationship between the specific heat capacities.