(Solved): Convert the following equation to Cartesian coordinates. Describe the resulting curve. r=8cos+5s ...

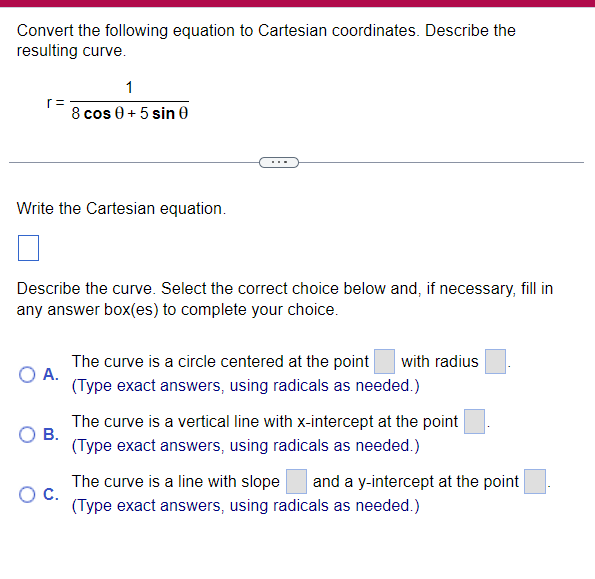
Convert the following equation to Cartesian coordinates. Describe the resulting curve. $$r=\frac{1}{8\cos ?+5\sin ?}$$ Write the Cartesian equation. Describe the curve. Select the correct choice below and, if necessary, fill in any answer box(es) to complete your choice. A. The curve is a circle centered at the point with radius (Type exact answers, using radicals as needed.) B. The curve is a vertical line with $$\mathrm{x}$$-intercept at the point (Type exact answers, using radicals as needed.) C. The curve is a line with slope and a y-intercept at the point (Type exact answers, using radicals as needed.)