(Solved): Consider two spin-half particles \\( \\alpha \\) and \\( \\beta \\) in a magnetic field \\( B \\hat ...

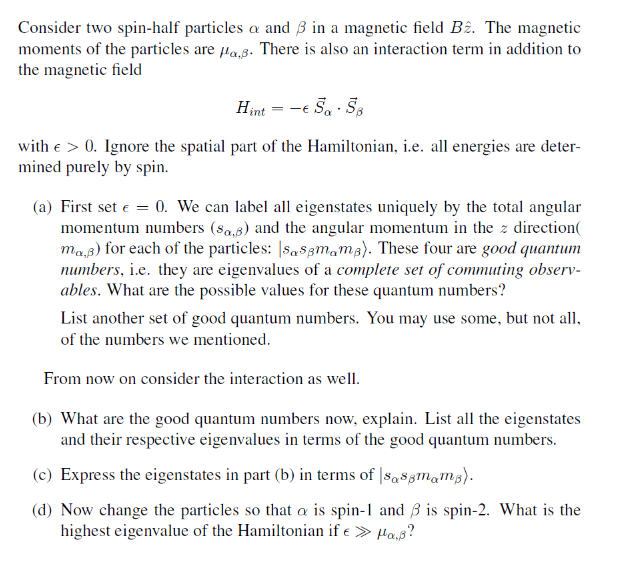
Consider two spin-half particles \\( \\alpha \\) and \\( \\beta \\) in a magnetic field \\( B \\hat{z} \\). The magnetic moments of the particles are \\( \\mu_{\\alpha, \\beta} \\). There is also an interaction term in addition to the magnetic field \\[ H_{\\text {int }}=-\\epsilon \\vec{S}_{\\alpha} \\cdot \\vec{S}_{\\beta} \\] with \\( \\epsilon>0 \\). Ignore the spatial part of the Hamiltonian, i.e. all energies are determined purely by spin. (a) First set \\( \\epsilon=0 \\). We can label all eigenstates uniquely by the total angular momentum numbers \\( \\left(s_{\\alpha, \\beta}\\right) \\) and the angular momentum in the \\( z \\) direction( \\( m_{\\alpha, \\beta} \\) ) for each of the particles: \\( \\left|s_{\\alpha} s_{\\beta} m_{\\alpha} m_{\\beta}\\right\\rangle \\). These four are good quantum numbers, i.e. they are eigenvalues of a complete set of commuting observables. What are the possible values for these quantum numbers? List another set of good quantum numbers. You may use some, but not all, of the numbers we mentioned. From now on consider the interaction as well. (b) What are the good quantum numbers now, explain. List all the eigenstates and their respective eigenvalues in terms of the good quantum numbers. (c) Express the eigenstates in part (b) in terms of \\( \\left|s_{\\alpha} s_{\\beta} m_{\\alpha} m_{\\beta}\\right\\rangle \\). (d) Now change the particles so that \\( \\alpha \\) is spin-1 and \\( \\beta \\) is spin-2. What is the highest eigenvalue of the Hamiltonian if \\( \\epsilon \\gg \\mu_{\\alpha, \\beta} \\) ?