Home /
Expert Answers /
Calculus /
consider-the-series-sum-n-1-infty-n-n-1-a-find-the-partial-sums-s-1-s-2-s-3-pa576
(Solved): Consider the series \sum_(n=1)^(\infty ) (n)/((n+1)!). (a) Find the partial sums s_(1),s_(2),s_(3 ...
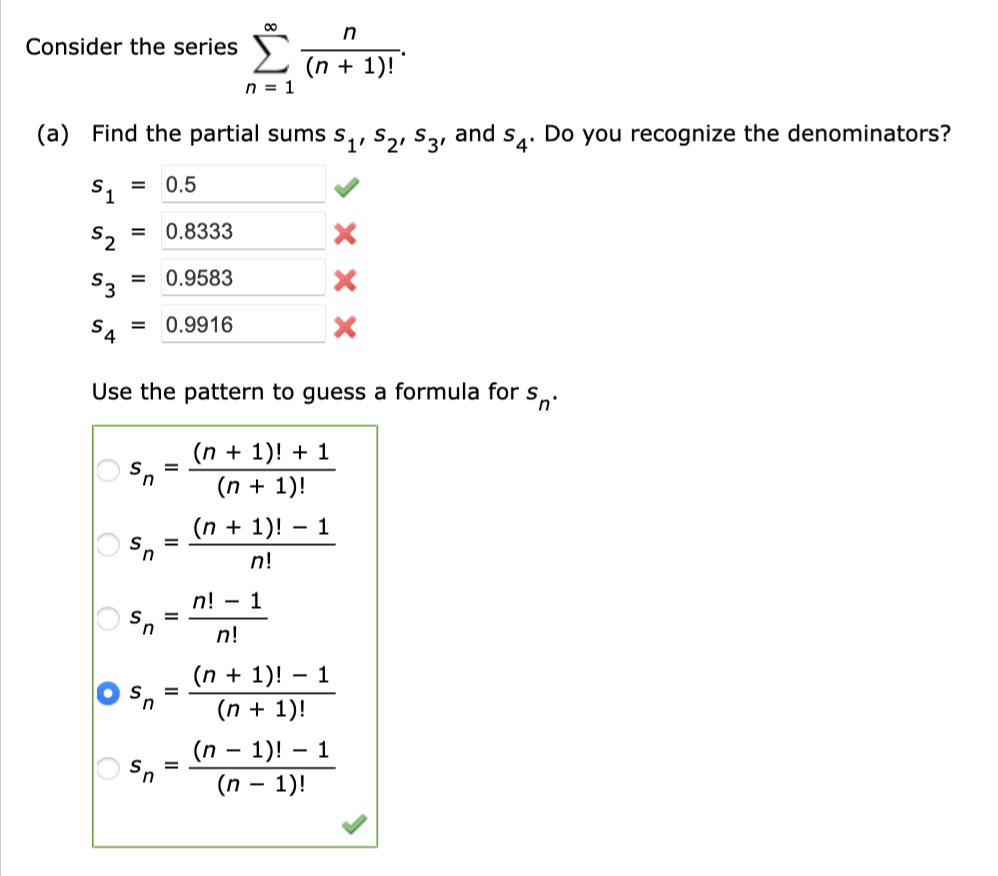
Consider the series
\sum_(n=1)^(\infty ) (n)/((n+1)!). (a) Find the partial sums
s_(1),s_(2),s_(3), and
s_(4). Do you recognize the denominators?
s_(1)=
s_(2)=
s_(3)=
s_(4)=Use the pattern to guess a formula for
s_(n).
s_(n)=((n+1)!+1)/((n+1)!)
s_(n)=((n+1)!-1)/(n!)
s_(n)=(n!-1)/(n!)
s_(n)=((n+1)!-1)/((n+1)!)
s_(n)=((n-1)!-1)/((n-1)!)Test the series for convergence or divergence using the Alternating Series Test.
\sum_(n=1)^(\infty ) ((-1)^(n-1))/(7+8n)Identify
b_(n).
(1+(-1)^(n))/(\sqrt(n)+4)Evaluate the following limit.
\lim_(n->\infty )b_(n)Since
\lim_(n->\infty )b_(n)=hat(?),0and
b_(n+1)<=hat(?)b_(n)for all
n, Test the series for convergence or divergence using the Alternating Series Test.
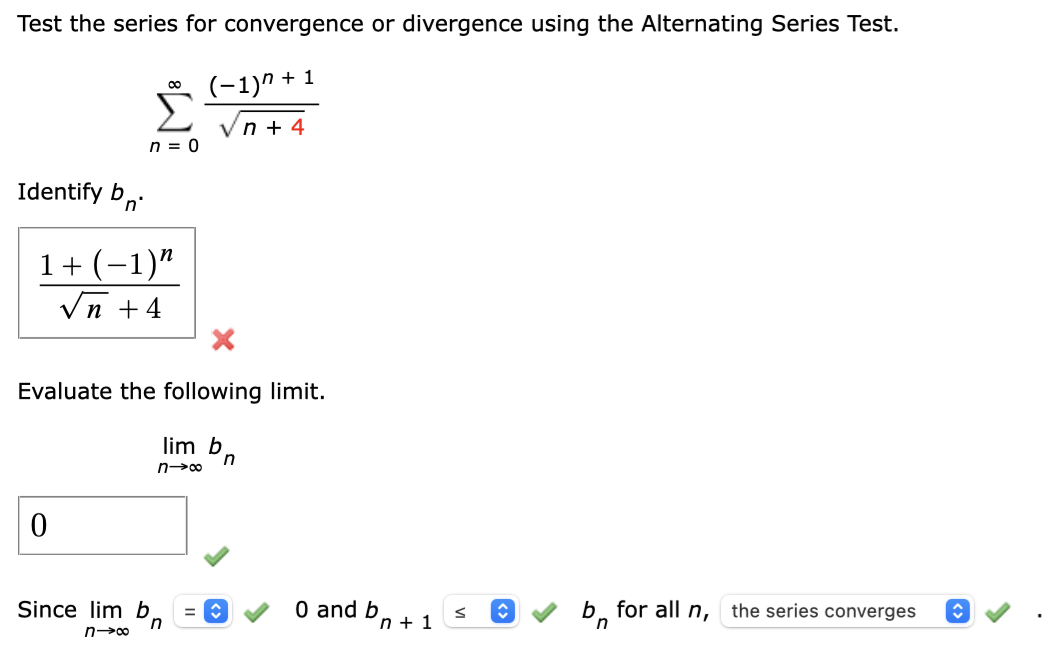
\sum_(n=0)^(\infty ) ((-1)^(n+1))/(\sqrt(n+4))Identify
b_(n).
(1+(-1)^(n))/(\sqrt(n)+4)Evaluate the following limit.
\lim_(n->\infty )b_(n)Since
\lim_(n->\infty )b_(n)=hat(?),0and
b_(n+1)<=hat(v)b_(n)for all
n,