(Solved): Consider the mixed Poisson distribution pn=0n!()neu ...

???????

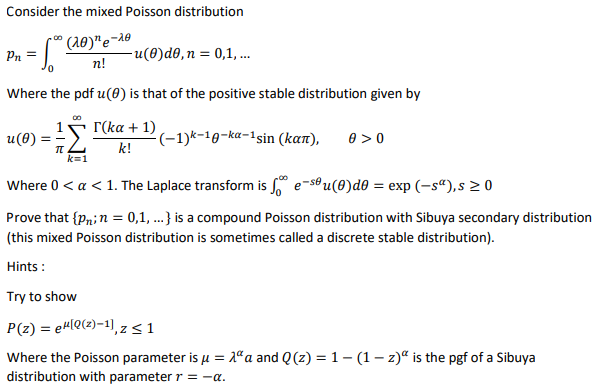
Consider the mixed Poisson distribution $p_n={?}_0^{?}\frac{(??{)}^n{e}^{???}}{n!}u(?)d?,n=0,1,\dots$ Where the pdf $u(?)$ is that of the positive stable distribution given by $u(?)=\frac{1}{?}{?}_{k=1}^{?}\frac{?(k?+1)}{k!}(?1{)}^{k?1}{?}^{?k??1}\sin (k??),?>0$ Where $0<?<1$. The Laplace transform is ${?}_0^{?}{e}^{?s?}u(?)d?=\exp \left(?s^{?}\right),s?0$ Prove that $\left\{p_n;n=0,1,\dots \right\}$ is a compound Poisson distribution with Sibuya secondary distribution (this mixed Poisson distribution is sometimes called a discrete stable distribution). Hints : Try to show $P(z)=e^{?[Q(z)?1]},z?1$ Where the Poisson parameter is $?={?}^{?}a$ and $Q(z)=1?(1?z{)}^{?}$ is the pgf of a Sibuya distribution with parameter $r=??$.