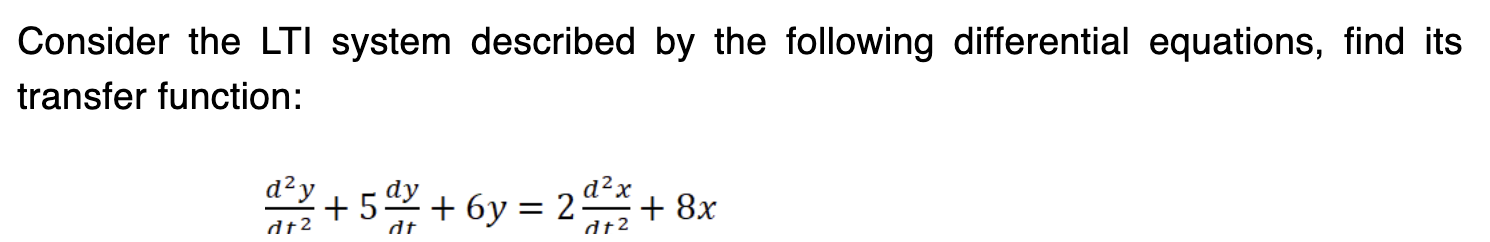Home /
Expert Answers /
Electrical Engineering /
consider-the-lti-system-described-by-the-following-differential-equations-find-its-transfer-functi-pa760
Expert Answer
To find the transfer function of the given linear time-invariant (LTI) system, we need to take the Laplace transform of the differential equations and rearrange the equations in terms of the Laplace variables. Let's assume that the input to the system is denoted by 'x(t)' and the output is denoted by 'y(t)'. The Laplace transform of a function 'f(t)' is denoted by 'F(s)'. The given differential equation can be written as: Taking the Laplace transform of both sides, we get: Here, y(0) and y'(0) represent the initial conditions of the output y(t). Rearranging the equation, we have: (s^2 + 5s + 6)Y(s) = (2s^2 + 8)X(s) + sy(0) + y'(0) - 5y(0) Dividing both sides by (s^2 + 5s + 6), we get: