(Solved): Consider the initial value problem for the function \( y \) \[ y^{\prime}-2 y^{1 / 3}=0, \quad y(0 ...

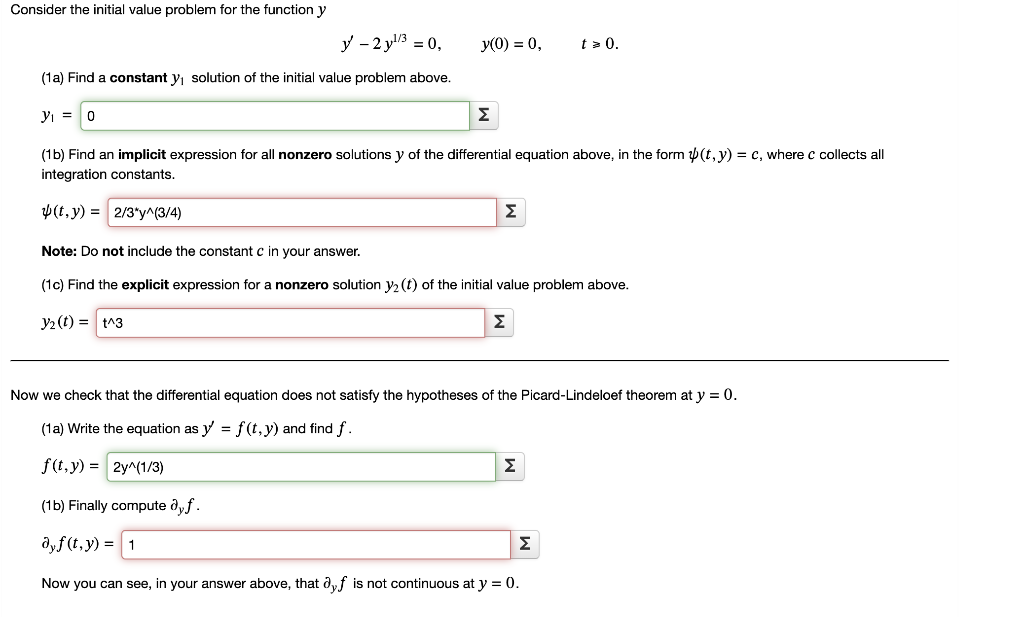
Consider the initial value problem for the function \( y \) \[ y^{\prime}-2 y^{1 / 3}=0, \quad y(0)=0, \quad t \geqslant 0 . \] (1a) Find a constant \( y_{1} \) solution of the initial value problem above. \[ y_{1}= \] (1b) Find an implicit expression for all nonzero solutions \( y \) of the differential equation above, in the form \( \psi(t, y)=c \), where \( c \) collects all integration constants. \[ \psi(t, y)= \] Note: Do not include the constant \( c \) in your answer. (1c) Find the explicit expression for a nonzero solution \( y_{2}(t) \) of the initial value problem above. \[ y_{2}(t) \] Now we check that the differential equation does not satisfy the hypotheses of the Picard-Lindeloef theorem at \( y=0 \). (1a) Write the equation as \( y^{\prime}=f(t, y) \) and find \( f \) \[ f(t, y)= \] (1b) Finally compute \( \partial_{y} f \). \[ \partial_{y} f(t, y)= \] Now you can see, in your answer above, that \( \partial_{y} f \) is not continuous at \( y=0 \).