(Solved): Consider the homogeneous linear equation Ax=0, (when the right-hand-side vector is the zero vector, ...

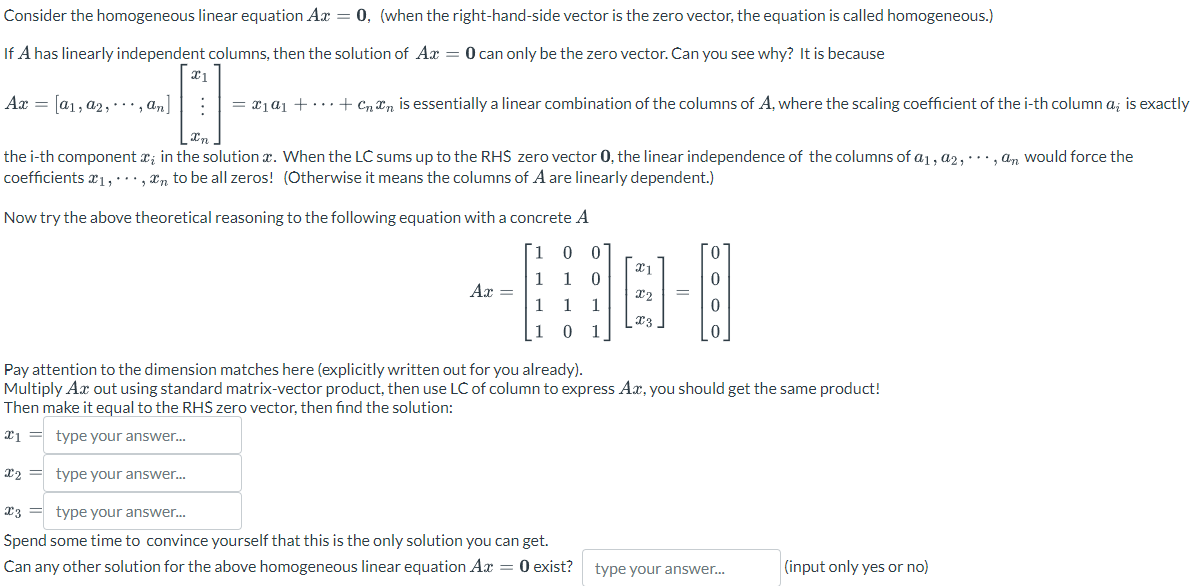
Consider the homogeneous linear equation $Ax=0$, (when the right-hand-side vector is the zero vector, the equation is called homogeneous.) If $A$ has linearly independent columns, then the solution of $Ax=0$ can only be the zero vector. Can you see why? It is because $Ax=\left[a_1,a_2,?,a_n\right]?\begin{array}{c}{x}_1\\ ?\\ x_n\end{array}?=x_1{a}_1+?+c_n{x}_n$ is essentially a linear combination of the columns of $A$, where the scaling coefficient of the $\mathrm{i}$-th column $a_i$ is exactly the i-th component $x_i$ in the solution $x$. When the LC sums up to the RHS zero vector $0$, the linear independence of the columns of $a_1,a_2,?,a_n$ would force the coefficients $x_1,?,x_n$ to be all zeros! (Otherwise it means the columns of $A$ are linearly dependent.) Now try the above theoretical reasoning to the following equation with a concrete $A$ $Ax=?\begin{array}{lll}1& 0& 0\\ 1& 1& 0\\ 1& 1& 1\\ 1& 0& 1\end{array}??\begin{array}{l}{x}_1\\ x_2\\ x_3\end{array}?=?\begin{array}{l}0\\ 0\\ 0\\ 0\end{array}?$ Pay attention to the dimension matches here (explicitly written out for you already). Multiply $Ax$ out using standard matrix-vector product, then use LC of column to express $Ax$, you should get the same product! Then make it equal to the RHS zero vector, then find the solution: $\begin{array}{l}{x}_1=\\ x_2=\\ x_3=\end{array}$ Spend some time to convince yourself that this is the only solution you can get. Can any other solution for the above homogeneous linear equation $Ax=0$ exist? (input only yes or no)