(Solved): Consider the generic feedback system shown in Figure 4 , where \( G(s) \) is the plant's transfer ...

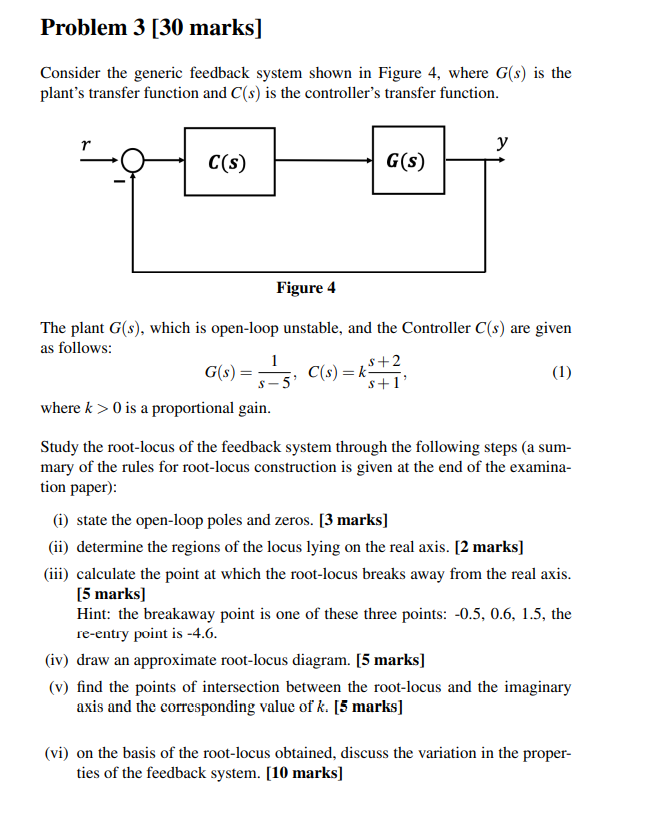
Consider the generic feedback system shown in Figure 4 , where \( G(s) \) is the plant's transfer function and \( C(s) \) is the controller's transfer function. The plant \( G(s) \), which is open-loop unstable, and the Controller \( C(s) \) are given as follows: \[ G(s)=\frac{1}{s-5}, \quad C(s)=k \frac{s+2}{s+1}, \] where \( k>0 \) is a proportional gain. Study the root-locus of the feedback system through the following steps (a summary of the rules for root-locus construction is given at the end of the examination paper): (i) state the open-loop poles and zeros. [3 marks] (ii) determine the regions of the locus lying on the real axis. [2 marks] (iii) calculate the point at which the root-locus breaks away from the real axis. [5 marks] Hint: the breakaway point is one of these three points: \( -0.5,0.6,1.5 \), the re-entry point is -4.6. (iv) draw an approximate root-locus diagram. [5 marks] (v) find the points of intersection between the root-locus and the imaginary axis and the corresponding value of \( k \) : [5 marks] (vi) on the basis of the root-locus obtained, discuss the variation in the properties of the feedback system. [10 marks]