(Solved): Consider the following system of linear inequalities, which as a set of simultaneous equations def ...

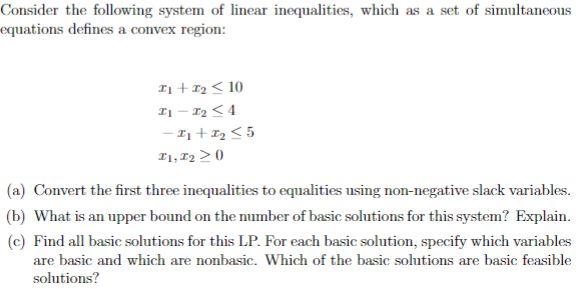
Consider the following system of linear inequalities, which as a set of simultaneous equations defines a convex region: \[ \begin{array}{l} x_{1}+x_{2} \leq 10 \\ x_{1}-x_{2} \leq 4 \\ -x_{1}+x_{2} \leq 5 \\ x_{1}, x_{2} \geq 0 \end{array} \] (a) Convert the first three inequalities to equalities using non-negative slack variables. (b) What is an upper bound on the number of basic solutions for this system? Explain. (c) Find all basic solutions for this LP. For each basic solution, specify which variables are basic and which are nonbasic. Which of the basic solutions are basic feasible solutions?