(Solved): Consider the following Stackelberg duopoly. Both firms produce a homogenous good. Firm 1 chooses h ...

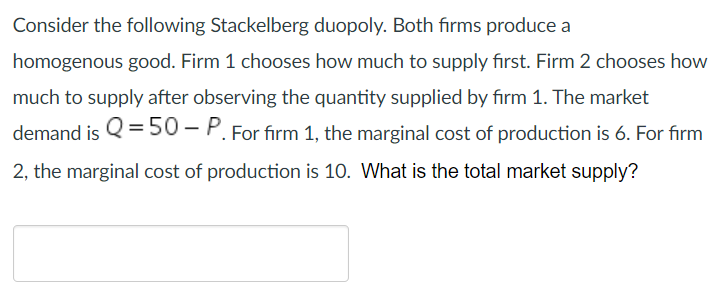
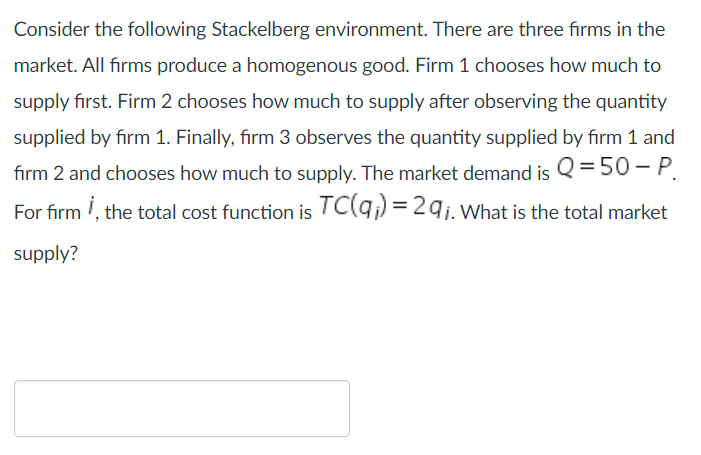
Consider the following Stackelberg duopoly. Both firms produce a homogenous good. Firm 1 chooses how much to supply first. Firm 2 chooses how much to supply after observing the quantity supplied by firm 1 . The market demand is \( Q=50-P \). For firm 1, the marginal cost of production is 6 . For firm 2, the marginal cost of production is 10 . What is the total market supply? Consider the following Stackelberg environment. There are three firms in the market. All firms produce a homogenous good. Firm 1 chooses how much to supply first. Firm 2 chooses how much to supply after observing the quantity supplied by firm 1. Finally, firm 3 observes the quantity supplied by firm 1 and firm 2 and chooses how much to supply. The market demand is \( Q=50-P \). For firm \( i \), the total cost function is \( T C\left(q_{i}\right)=2 q_{i} \). What is the total market supply? 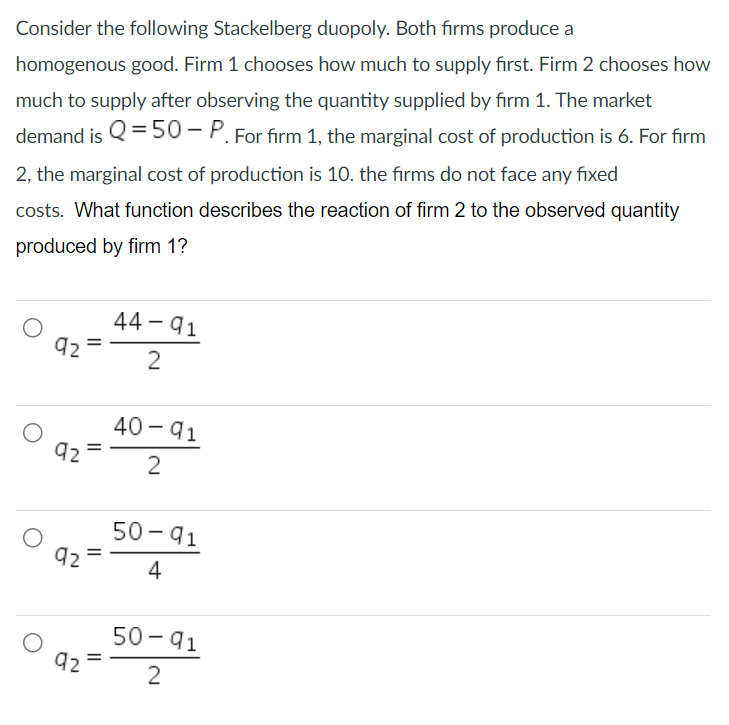Consider the following Stackelberg duopoly. Both firms produce a homogenous good. Firm 1 chooses how much to supply first. Firm 2 chooses how much to supply after observing the quantity supplied by firm 1 . The market demand is \( Q=50-P \). For firm 1, the marginal cost of production is 6 . For firm 2, the marginal cost of production is 10. the firms do not face any fixed costs. What function describes the reaction of firm 2 to the observed quantity produced by firm 1 ? \[ q_{2}=\frac{44-q_{1}}{2} \] \[ q_{2}=\frac{40-q_{1}}{2} \] \[ q_{2}=\frac{50-q_{1}}{4} \] \[ q_{2}=\frac{50-q_{1}}{2} \] Consider the following Stackelberg duopoly. Both firms produce differentiated goods. For form \( i \), the demand is \( q_{i}=10-p_{i}+\frac{1}{2} p_{j} \). Firm 1 chooses the price first. Firm 2 chooses the price after observing the choice of firm 1. For firm \( i \) , the total cost function is \( T C\left(q_{i}\right)=2 q_{i} \). What function describes the reaction of firm 2 to the choices of firm 1 ? \[ p_{2}=12+\frac{1}{2} p_{1} \] \[ p_{2}=24+\frac{1}{2} p_{1} \] \[ p_{2}=6+\frac{1}{4} p_{1} \] \[ p_{2}=6+\frac{1}{8} p_{1} \]