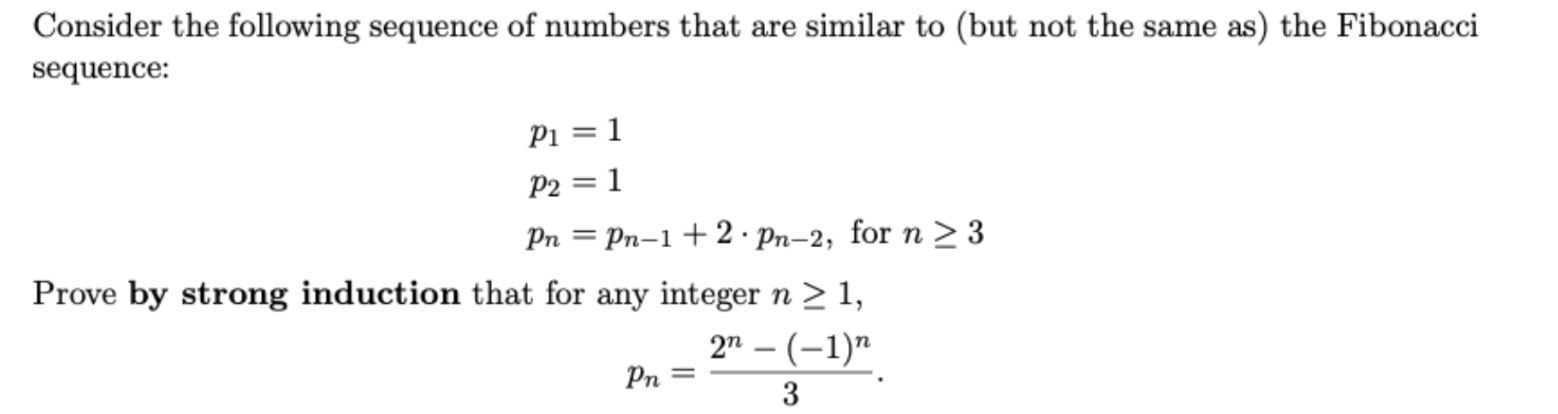(Solved): Consider the following sequence of numbers that are similar to (but not the same as) the Fibonacci ...

Consider the following sequence of numbers that are similar to (but not the same as) the Fibonacci sequence: \[ \begin{array}{l} p_{1}=1 \\ p_{2}=1 \\ p_{n}=p_{n-1}+2 \cdot p_{n-2}, \text { for } n \geq 3 \end{array} \] Prove by strong induction that for any integer \( n \geq 1 \), \[ p_{n}=\frac{2^{n}-(-1)^{n}}{3} \text {. } \]