(Solved): Consider the following numerical example of maximising social welfare. \( A \) and \( B \) must spl ...

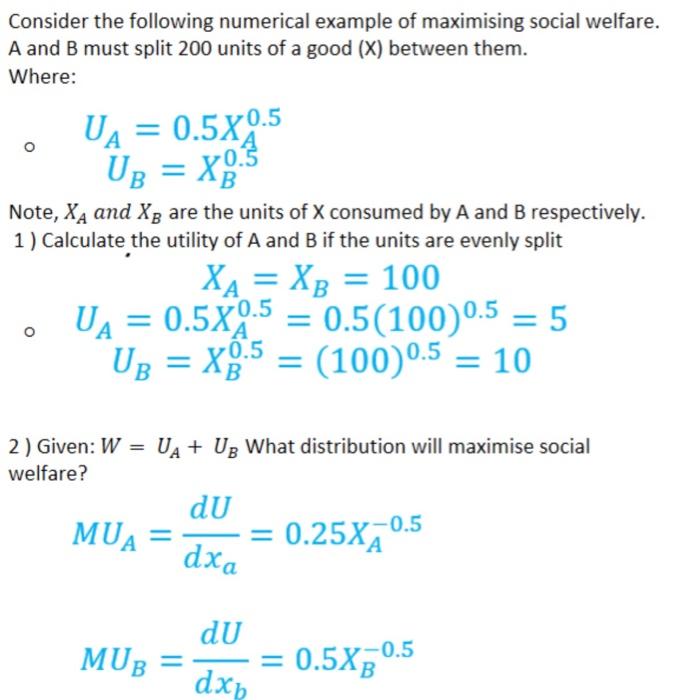
Consider the following numerical example of maximising social welfare. \( A \) and \( B \) must split 200 units of a good \( (X) \) between them. Where: \[ \begin{array}{c} U_{A}=0.5 X_{A}^{0.5} \\ U_{B}=X_{B}^{0.5} \end{array} \] Note, \( X_{A} \) and \( X_{B} \) are the units of \( X \) consumed by \( A \) and \( B \) respectively. 1) Calculate the utility of \( A \) and \( B \) if the units are evenly split \[ X_{A}=X_{B}=100 \] \( \circ \quad U_{A}=0.5 X_{A}^{0.5}=0.5(100)^{0.5}=5 \) \( U_{B}=X_{B}^{0.5}=(100)^{0.5}=10 \) 2) Given: \( W=U_{A}+U_{B} \) What distribution will maximise social welfare? \[ M U_{A}=\frac{d U}{d x_{a}}=0.25 X_{A}^{-0.5} \] \[ M U_{B}=\frac{d U}{d x_{b}}=0.5 X_{B}^{-0.5} \]