(Solved): Consider the following linear transformation and basis. \[ T: R^{2} \rightarrow R^{2}, T(x, y)=(x- ...

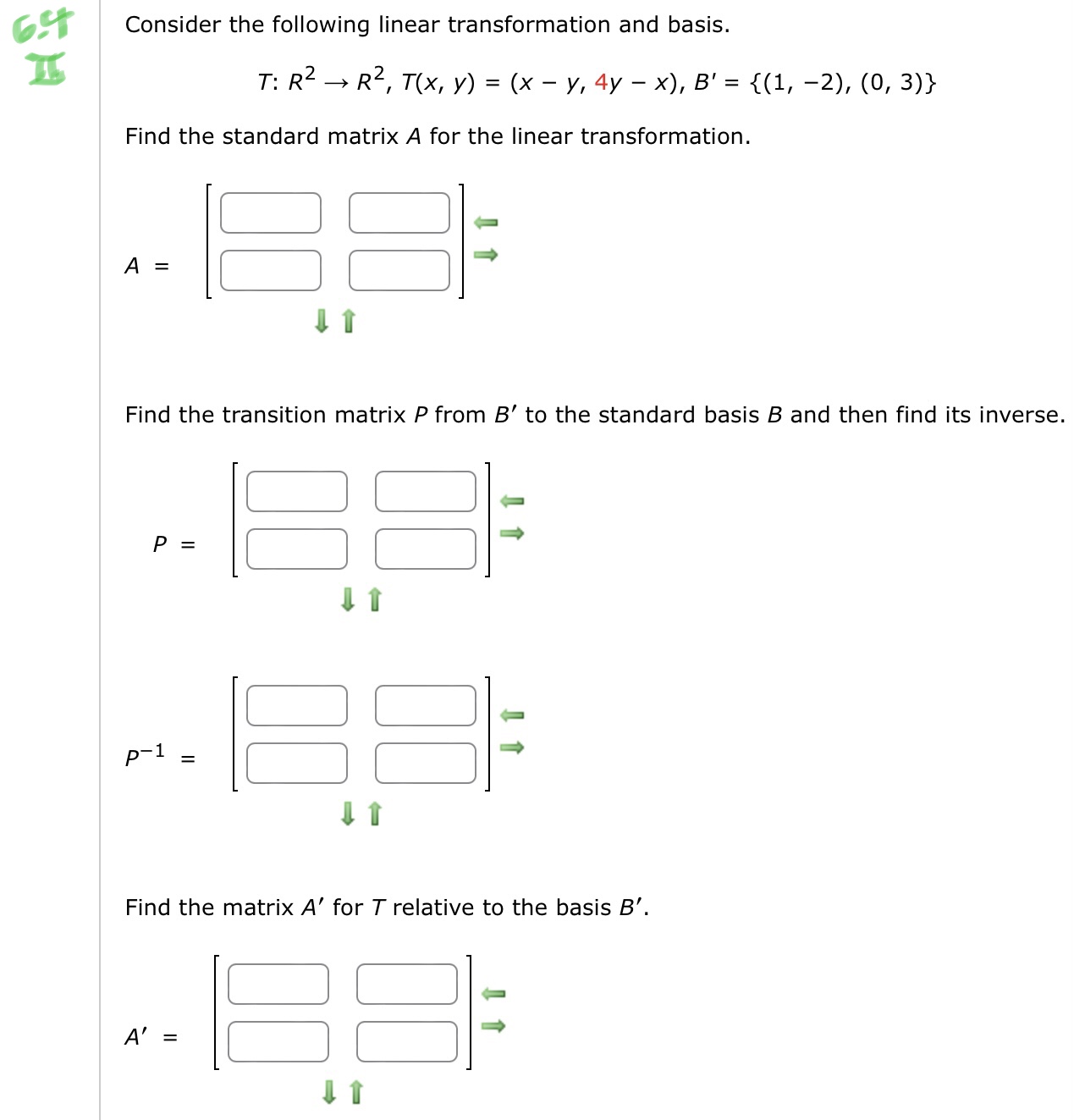
Consider the following linear transformation and basis. \[ T: R^{2} \rightarrow R^{2}, T(x, y)=(x-y, 4 y-x), B^{\prime}=\{(1,-2),(0,3)\} \] Find the standard matrix \( A \) for the linear transformation. \[ A=\left[\begin{array}{ll} \\ \Downarrow \end{array}\right] \Rightarrow \] Find the transition matrix \( P \) from \( B^{\prime} \) to the standard basis \( B \) and then find its inverse. \[ \begin{array}{l} P=[\quad] \Rightarrow \\ \Downarrow \Uparrow \\ P^{-1}=[\quad] \Rightarrow \\ \Downarrow \Uparrow \\ \end{array} \] Find the matrix \( A^{\prime} \) for \( T \) relative to the basis \( B^{\prime} \). \[ A^{\prime}=\left[\begin{array}{ll} \\ \Downarrow \mathbb{\|} \end{array}\right] \Rightarrow \]