(Solved): Consider the enzyme catalyzed liquid reaction \( S \rightarrow P \) run in a steady isothermal CST ...

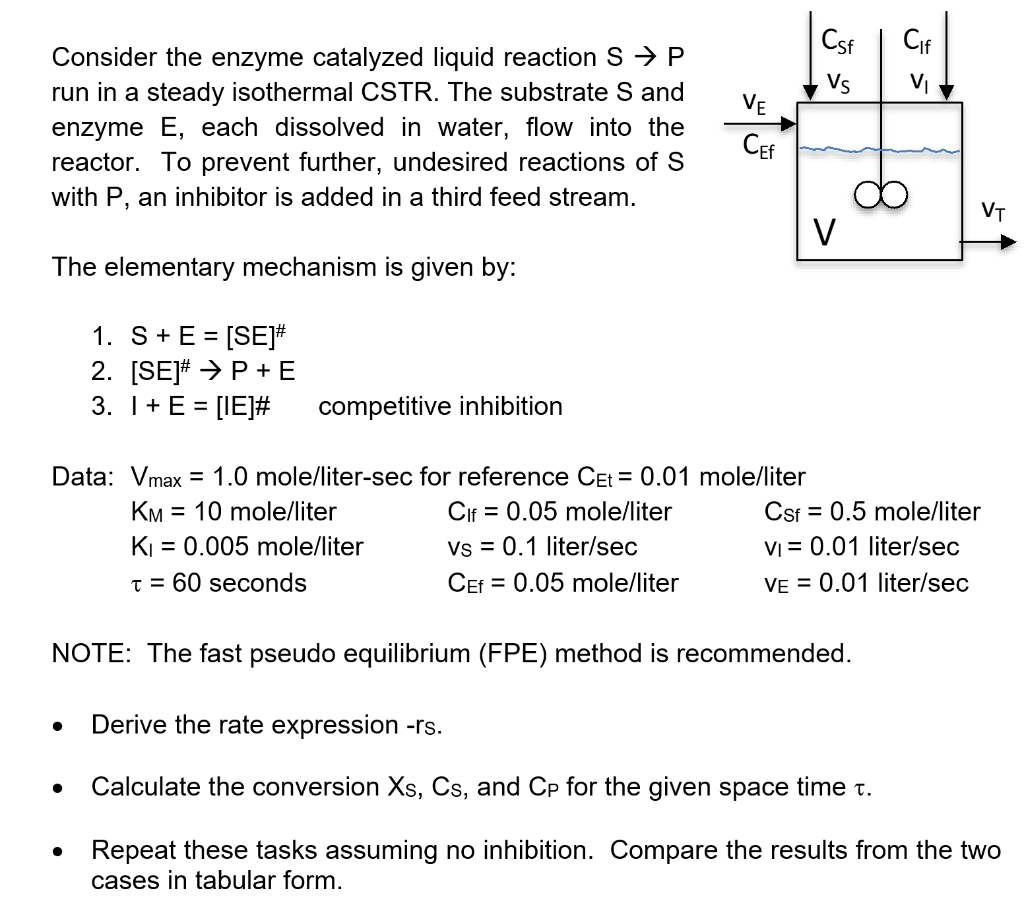
Consider the enzyme catalyzed liquid reaction \( S \rightarrow P \) run in a steady isothermal CSTR. The substrate \( S \) and enzyme \( E \), each dissolved in water, flow into the reactor. To prevent further, undesired reactions of \( S \) with \( \mathrm{P} \), an inhibitor is added in a third feed stream. The elementary mechanism is given by: 1. \( S+E=[S E]^{\#} \) 2. \( [\mathrm{SE}]^{\#} \rightarrow \mathrm{P}+\mathrm{E} \) 3. \( I+E=[I E] \# \quad \) competitive inhibition Data: \( V_{\max }=1.0 \) mole/liter-sec for reference \( C_{E t}=0.01 \) mole/liter \( \begin{array}{lll}\mathrm{K}_{\mathrm{M}}=10 \text { mole/liter } & \mathrm{C}_{\mathrm{If}}=0.05 \mathrm{~mole} / \mathrm{liter} & \mathrm{C}_{\mathrm{Sf}}=0.5 \mathrm{~mole} / \mathrm{liter} \\ \mathrm{K}_{\mathrm{I}}=0.005 \text { mole/liter } & \mathrm{V}_{\mathrm{S}}=0.1 \mathrm{liter} / \mathrm{sec} & \mathrm{V}_{\mathrm{I}}=0.01 \mathrm{liter} / \mathrm{sec} \\ \tau=60 \mathrm{~seconds} & \mathrm{C}_{\mathrm{Ef}}=0.05 \mathrm{~mole} / \mathrm{liter} & \mathrm{V}_{\mathrm{E}}=0.01 \mathrm{liter} / \mathrm{sec}\end{array} \) NOTE: The fast pseudo equilibrium (FPE) method is recommended. - Derive the rate expression -rs. - Calculate the conversion \( \mathrm{X}_{\mathrm{s}}, \mathrm{C} \), and \( \mathrm{CP} \) for the given space time \( \tau \). - Repeat these tasks assuming no inhibition. Compare the results from the two