Home /
Expert Answers /
Calculus /
consider-the-continuous-function-f-x-y-x-2-y-2-x-2-y-2-2-defined-for-all-x-y-in-0-pa635
(Solved): Consider the continuous function f(x,y)=(x^(2)-y^(2))/((x^(2)+y^(2))^(2)) defined for all (x,y)in(0, ...
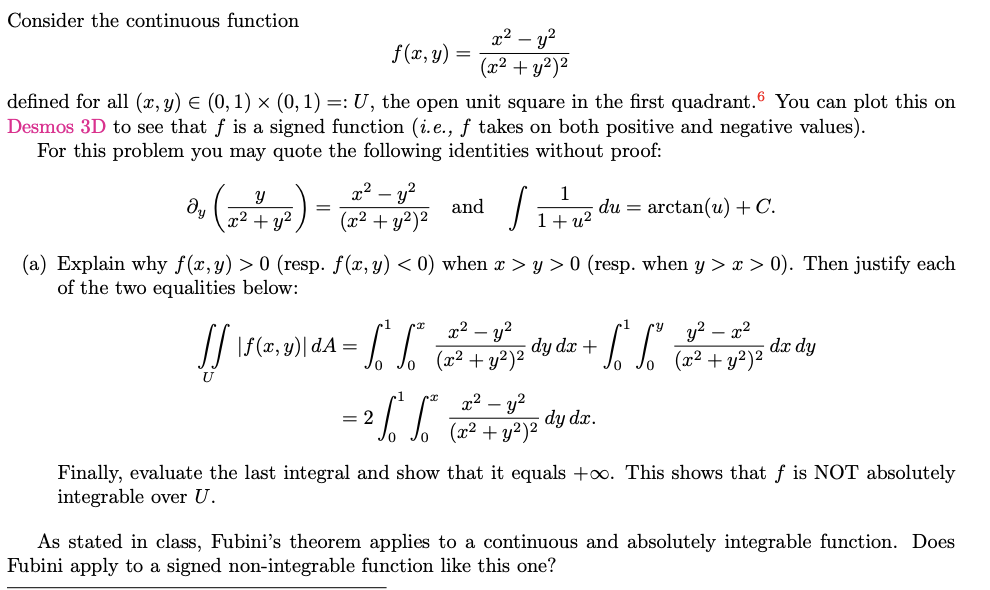
Consider the continuous function
f(x,y)=(x^(2)-y^(2))/((x^(2)+y^(2))^(2))
defined for all (x,y)in(0,1)\times (0,1)=:U, the open unit square in the first quadrant. ^(()6) You can plot this on
Desmos 3D to see that ff takes on both positive and negative valuesdel_(y)((y)/(x^(2)+y^(2)))=(x^(2)-y^(2))/((x^(2)+y^(2))^(2)), and ,\int (1)/(1+u^(2))du=arctan(u)+C
(a) Explain why f(x,y)>0f(x,y)<0 x>y>0y>x>0 ?_(U)|f(x,y)|dA=\int_0^1 \int_0^x (x^(2)-y^(2))/((x^(2)+y^(2))^(2))dydx+\int_0^1 \int_0^y (y^(2)-x^(2))/((x^(2)+y^(2))^(2))dxdy
=2\int_0^1 \int_0^x (x^(2)-y^(2))/((x^(2)+y^(2))^(2))dydx
Finally, evaluate the last integral and show that it equals +\infty . This shows that f is NOT absolutely
integrable over U.
As stated in class, Fubini's theorem applies to a continuous and absolutely integrable function. Does
Fubini apply to a signed non-integrable function like this one?