Home /
Expert Answers /
Mechanical Engineering /
consider-an-mdof-system-shown-in-the-figure-below-the-system-parameters-are-ml-m2-1kg-m3-0-4-pa920
(Solved): Consider an MDOF system shown in the figure below. The system parameters are ml=m2=1kg;m3=0.4 ...
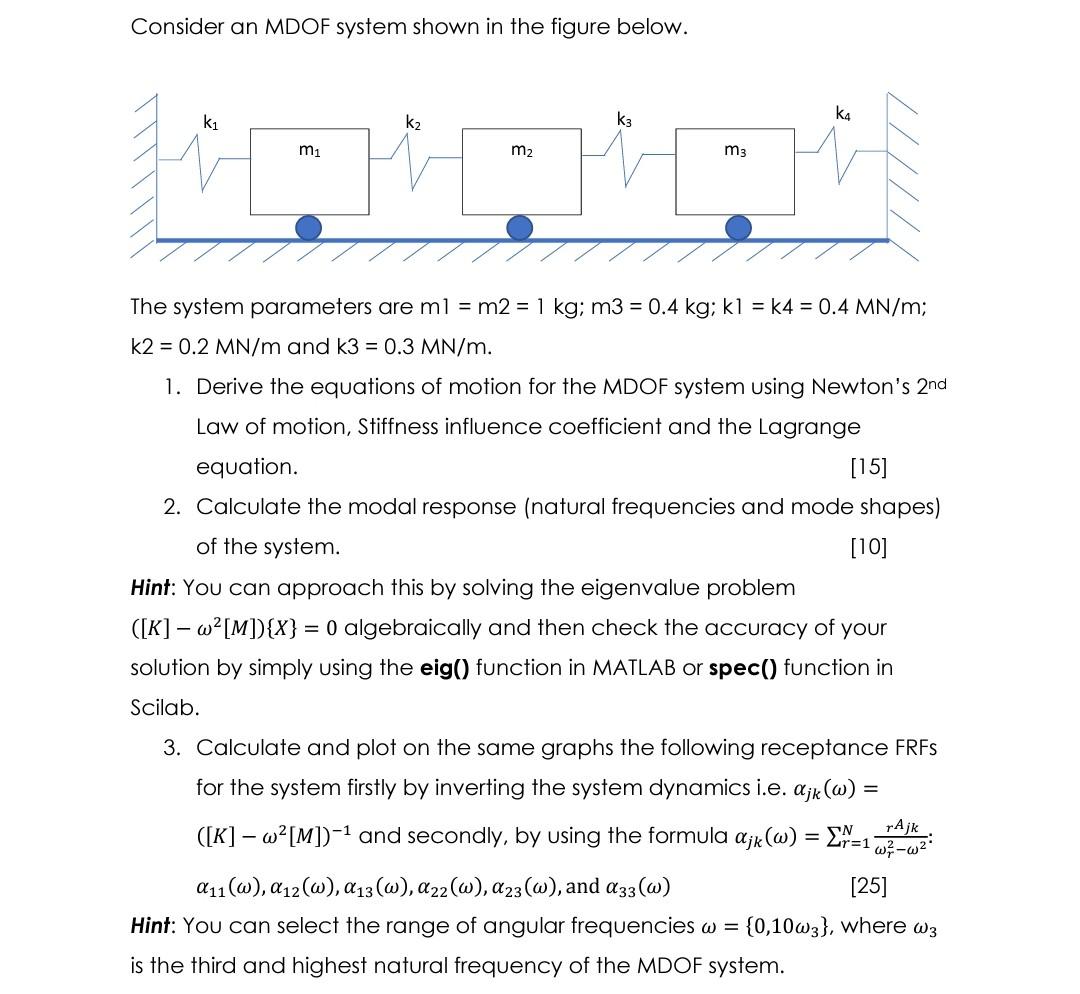
Consider an MDOF system shown in the figure below. The system parameters are ; and . 1. Derive the equations of motion for the MDOF system using Newton's Law of motion, Stiffness influence coefficient and the Lagrange equation. 2. Calculate the modal response (natural frequencies and mode shapes) of the system. Hint: You can approach this by solving the eigenvalue problem algebraically and then check the accuracy of your solution by simply using the eig() function in MATLAB or spec() function in Scilab. 3. Calculate and plot on the same graphs the following receptance FRFs for the system firstly by inverting the system dynamics i.e. and secondly, by using the formula , and Hint: You can select the range of angular frequencies , where is the third and highest natural frequency of the MDOF system.
Expert Answer
1. Deriving Equations of Motion: Using Newton's second law of motion, stiffness influence coefficients, and the Lagrange equation, we can derive the equations of motion for the MDOF system.Newton's Second Law: The equation of motion for the MDOF system can be written as: [M]{¨x} + [K]{x} = {F}Mass Matrix [M]: [M] = [m] = diag(m1, m2, m3) [m] = [1, 0, 0; 0, 1, 0; 0, 0, 0.4]Stiffness Matrix [K]: [K] = [ kl+k2, -kl, 0 ] [ -kl, kl+k3, -kl ] [ 0, -kl, kl+k4 ] [ kl = k4 = 0.4 MN/m, k2 = 0.2 MN/m, k3 = 0.3 MN/m ]Lagrange Equation: ?T/?q - d/dt(?T/?(¨q)) = ?U/?qKinetic Energy (T): T = (1/2) * {q}^T * [M] * {¨q}