(Solved): Consider a sphere of radius \\( R \\) subjected to diametral compression (Fig. 1.35). Let \\( \ ...

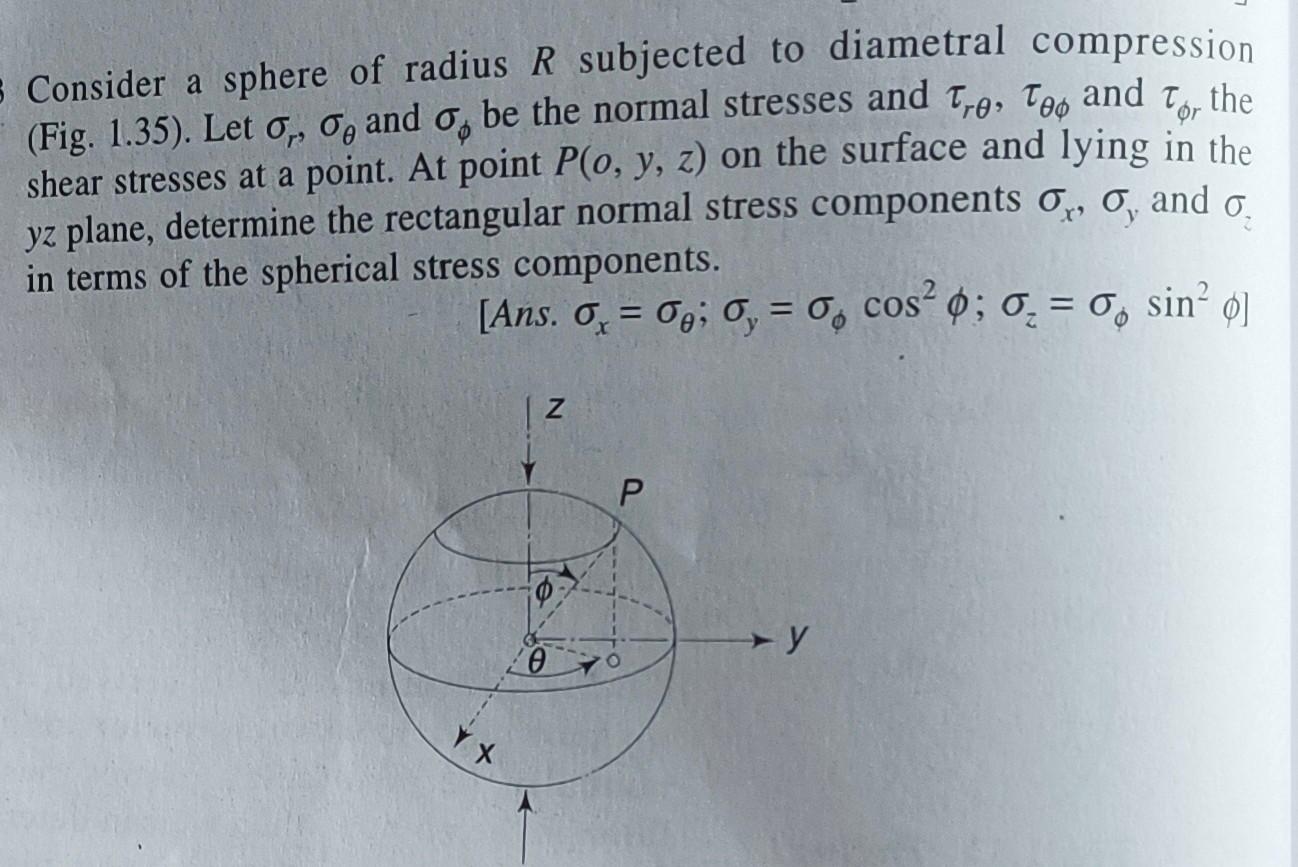
Consider a sphere of radius \\( R \\) subjected to diametral compression (Fig. 1.35). Let \\( \\sigma_{r}, \\sigma_{\\theta} \\) and \\( \\sigma_{\\phi} \\) be the normal stresses and \\( \\tau_{r \\theta}, \\tau_{\\theta \\phi} \\) and \\( \\tau_{\\phi r} \\) the shear stresses at a point. At point \\( P(o, y, z) \\) on the surface and lying in the \\( y z \\) plane, determine the rectangular normal stress components \\( \\sigma_{x}, \\sigma_{y} \\) and \\( \\sigma_{z} \\) in terms of the spherical stress components. \\( \\left[\\right. \\) Ans. \\( \\left.\\sigma_{x}=\\sigma_{\\theta} ; \\sigma_{y}=\\sigma_{\\phi} \\cos ^{2} \\phi ; \\sigma_{z}=\\sigma_{\\phi} \\sin ^{2} \\phi\\right] \\)