(Solved): Consider a set of samples {x1,x2,,xN} that are independent and identically distributed ...

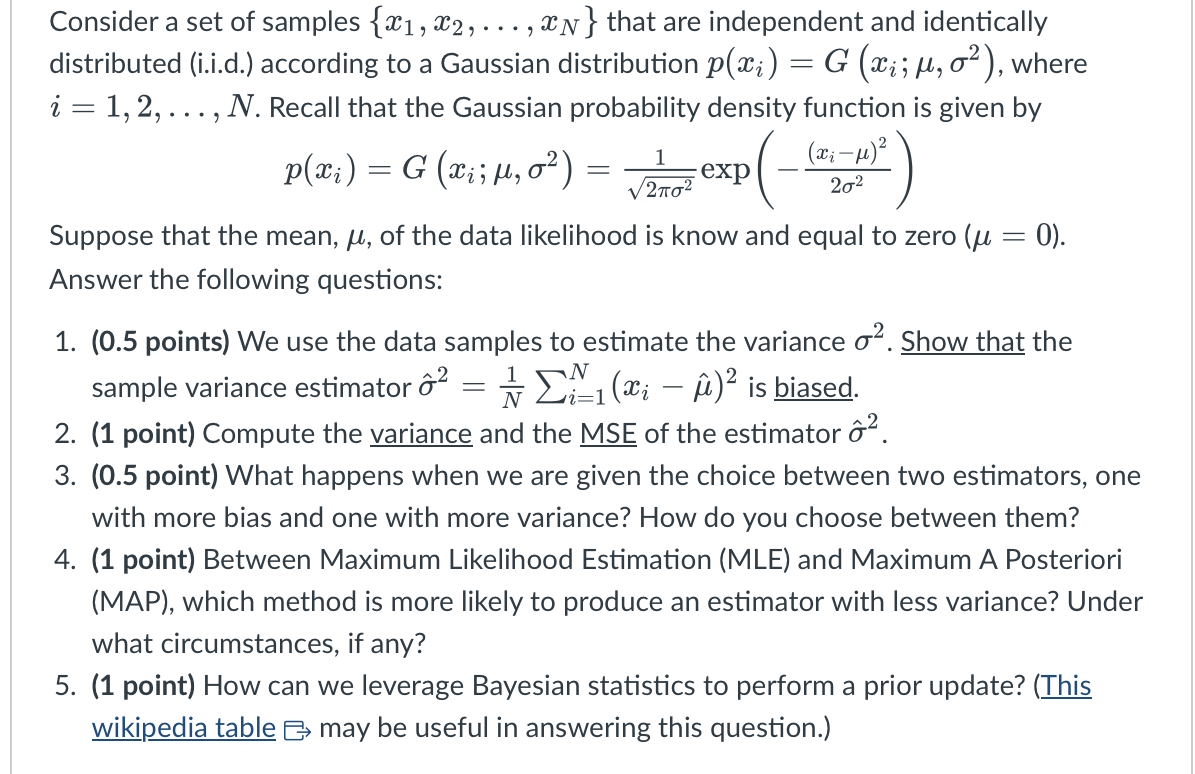
Consider a set of samples $\left\{x_1,x_2,\dots ,x_N\right\}$ that are independent and identically distributed (i.i.d.) according to a Gaussian distribution $p\left(x_i\right)=G\left(x_i;?,{?}^2\right)$, where $i=1,2,\dots ,N$. Recall that the Gaussian probability density function is given by $p\left(x_i\right)=G\left(x_i;?,{?}^2\right)=\frac{1}{\sqrt{2?{?}^2}}\exp \left(?\frac{{\left(x_i??\right)}^2}{2{?}^2}\right)$ Suppose that the mean, $?$, of the data likelihood is know and equal to zero $(?=0)$. Answer the following questions: 1. (0.5 points) We use the data samples to estimate the variance ${?}^2$. Show that the sample variance estimator ${\widehat{?}}^2=\frac{1}{N}{?}_{i=1}^N{\left(x_i?\widehat{?}\right)}^2$ is biased. 2. (1 point) Compute the variance and the MSE of the estimator ${\widehat{?}}^2$. 3. (0.5 point) What happens when we are given the choice between two estimators, one with more bias and one with more variance? How do you choose between them? 4. (1 point) Between Maximum Likelihood Estimation (MLE) and Maximum A Posteriori (MAP), which method is more likely to produce an estimator with less variance? Unde what circumstances, if any? 5. (1 point) How can we leverage Bayesian statistics to perform a prior update? (This wikipedia table $?$ may be useful in answering this question.)