(Solved): Consider a mechanical seismometer shown below. This is a base-excited system whose equation of mot ...

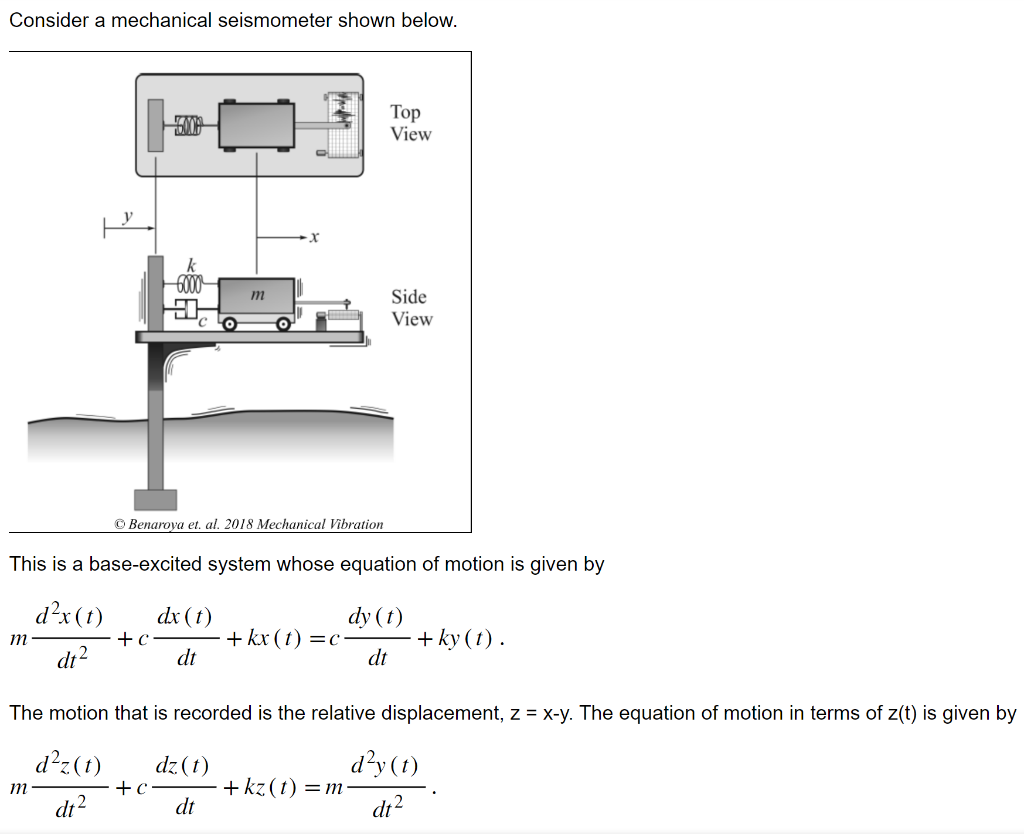
Consider a mechanical seismometer shown below. This is a base-excited system whose equation of motion is given by \[ m \frac{d^{2} x(t)}{d t^{2}}+c \frac{d x(t)}{d t}+k x(t)=c \frac{d y(t)}{d t}+k y(t) . \] The motion that is recorded is the relative displacement, \( z=x-y \). The equation of motion in terms of \( z(t) \) is given by \[ m \frac{d^{2} z(t)}{d t^{2}}+c \frac{d z(t)}{d t}+k z(t)=m \frac{d^{2} y(t)}{d t^{2}} \] \[ z_{s s}(t)=Z \cos (\omega t+\phi) \] where \[ Z=Y\left|\frac{-m \omega^{2}}{k-m \omega^{2}+i c \omega}\right|=Y \frac{m \omega^{2}}{\sqrt{\left(k-m \omega^{2}\right)^{2}+(c \omega)^{2}}} \] The steady-state relative displacement is given by \[ \begin{array}{l} =Y \frac{\omega^{2}}{\sqrt{\left(\omega_{n}^{2}-\omega^{2}\right)^{2}+\left(2 \zeta \omega \omega_{n} \omega\right)^{2}}}=Y \frac{r^{2}}{\sqrt{\left(1-r^{2}\right)^{2}+(2 \zeta r)^{2}}} \\ \text { and } \\ \phi=\angle \frac{-1}{k-m \omega^{2}+i c \omega}=\angle \frac{-1}{\omega_{n}^{2}-\omega^{2}+i 2 \zeta \omega_{n} \omega}=\angle \frac{-1}{1-r^{2}+i 2 \zeta r} \end{array} \] The figure below shows the magnification ratio, \( Z / Y \), as a function of frequency ratio, \( r= \) wf/wn. The maximum steady-state amplitude and the frequency when it occurs are \[ Z_{\text {res }}=Y \frac{1}{2 \zeta \sqrt{1-\zeta^{2}}} \text { and } \omega{ }_{r e s}=\omega \frac{1}{n \sqrt{1-2 \zeta^{2}}} \text {. } \] natural frequency so that the amplitude of \( z(t) \) is also the amplitude of \( y(t) \). Such seismometers (with low natural frequency) is called the long-period seismometer. have the gain of 1. If not, the amplitude of the recorded motion, \( Z \), may not be close to the amplitude of the actual ground motion, \( Y \). What is the amplitude of the recorded motion, \( Z \), when the input frequency is 10 ? Use 3 significant figures.