(Solved): Consider a linear time-invariant (LTI) system with input \( x(t) \) and output \( y(t) \) that is ...

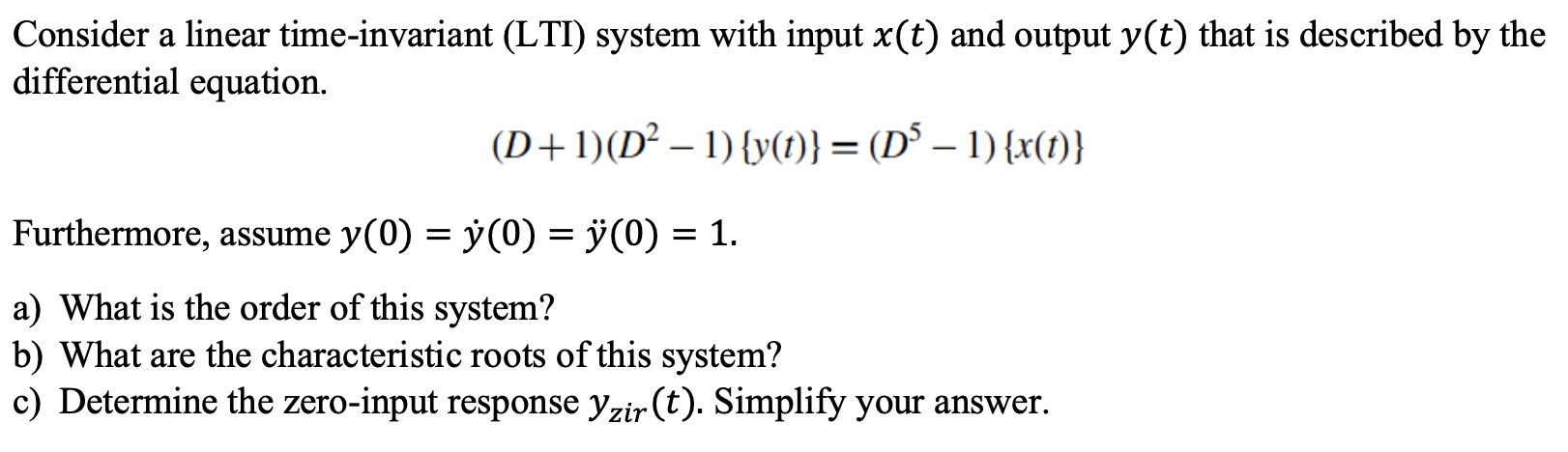
Consider a linear time-invariant (LTI) system with input \( x(t) \) and output \( y(t) \) that is described by the differential equation. \[ (D+1)\left(D^{2}-1\right)\{y(t)\}=\left(D^{5}-1\right)\{x(t)\} \] Furthermore, assume \( y(0)=\dot{y}(0)=\ddot{y}(0)=1 \). a) What is the order of this system? b) What are the characteristic roots of this system? c) Determine the zero-input response \( y_{z i r}(t) \). Simplify your answer.