(Solved): Compute the product using the methods below. If a product is undefined, explain why. a. The defini ...

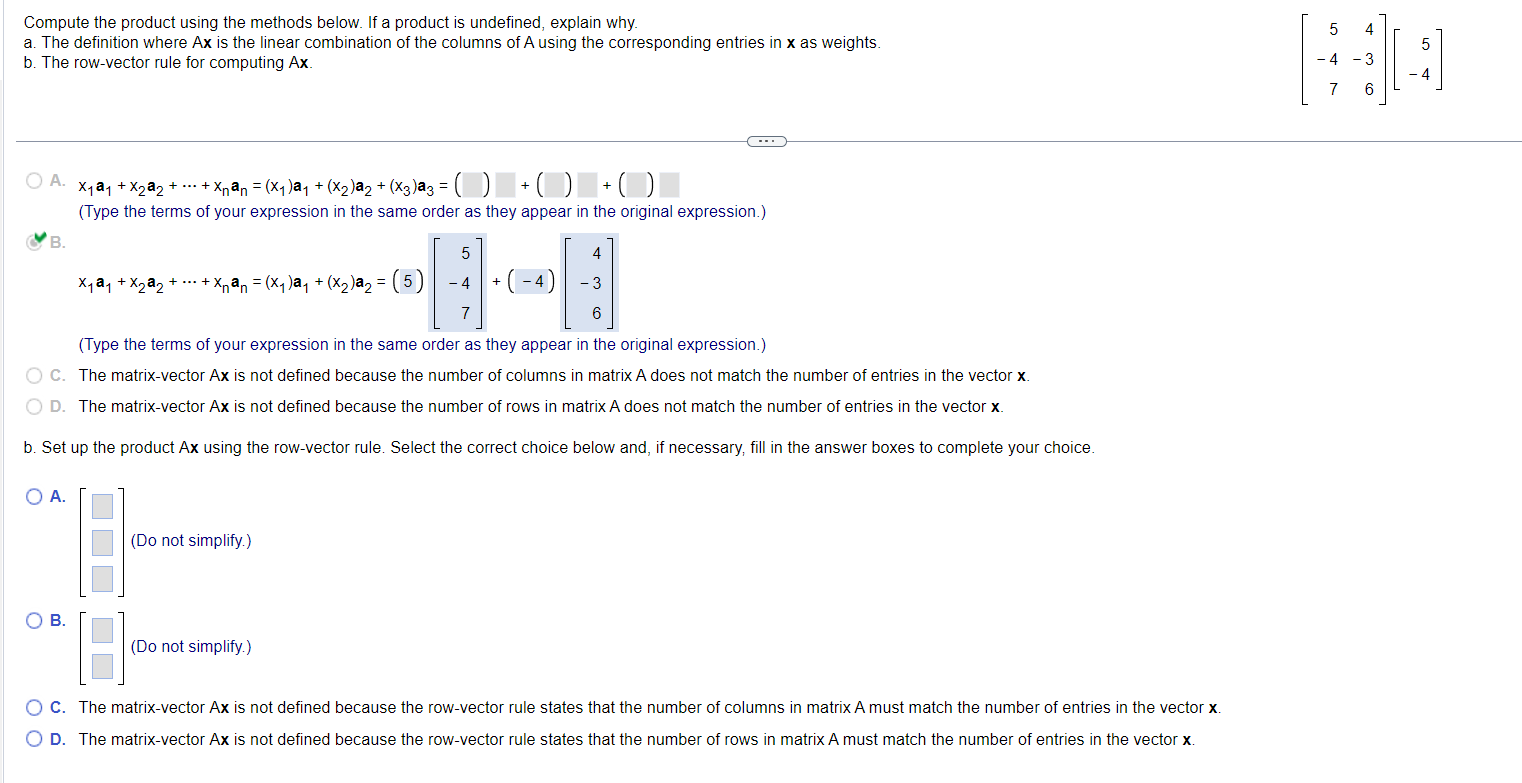
Compute the product using the methods below. If a product is undefined, explain why. a. The definition where Ax is the linear combination of the columns of A using the corresponding entries in \( \mathbf{x} \) as weights. b. The row-vector rule for computing \( \mathrm{Ax} \). \[ \left[\begin{array}{rr} 5 & 4 \\ -4 & -3 \\ 7 & 6 \end{array}\right]\left[\begin{array}{r} 5 \\ -4 \end{array}\right] \] A. \( x_{1} a_{1}+x_{2} a_{2}+\cdots+x_{n} a_{n}=\left(x_{1}\right) a_{1}+\left(x_{2}\right) \mathbf{a}_{2}+\left(x_{3}\right) \mathbf{a}_{3}=()+1 \) (Type the terms of your expression in the same order as they appear in the original expression.) B. \[ x_{1} a_{1}+x_{2} a_{2}+\cdots+x_{n} a_{n}=\left(x_{1}\right) a_{1}+\left(x_{2}\right) \mathbf{a}_{2}=(5)\left[\begin{array}{r} 5 \\ -4 \\ 7 \end{array}\right]+(-4)\left[\begin{array}{r} 4 \\ -3 \\ 6 \end{array}\right] \] (Type the terms of your expression in the same order as they appear in the original expression.) C. The matrix-vector \( \mathrm{Ax} \) is not defined because the number of columns in matrix \( \mathrm{A} \) does not match the number of entries in the vector \( \mathbf{x} \). D. The matrix-vector Ax is not defined because the number of rows in matrix A does not match the number of entries in the vector \( \mathbf{x} \). b. Set up the product Ax using the row-vector rule. Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice. A. \( ] \) (Do not simplify.) B. [] (Do not simplify.) C. The matrix-vector \( A \mathbf{x} \) is not defined because the row-vector rule states that the number of columns in matrix A must match the number of entries in the vector \( \mathbf{x} \). D. The matrix-vector Ax is not defined because the row-vector rule states that the number of rows in matrix \( A \) must match the number of entries in the vector \( \mathbf{x} \).