Home /
Expert Answers /
Calculus /
compute-the-flux-of-the-vector-field-vec-f-4x-2-y-2-zvec-k-through-the-surface-s-which-is-the-co-pa495
(Solved): Compute the flux of the vector field vec(F)=4x^(2)y^(2)zvec(k) through the surface S which is the co ...
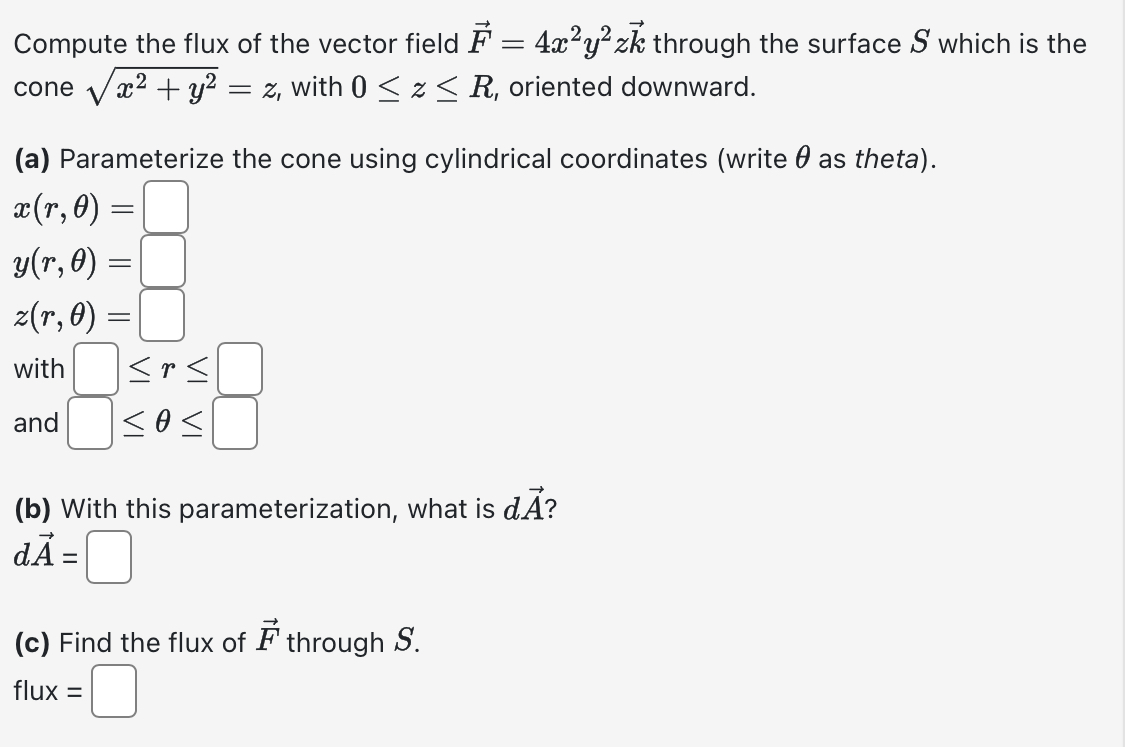
Compute the flux of the vector field
vec(F)=4x^(2)y^(2)zvec(k)through the surface
Swhich is the cone
\sqrt(x^(2)+y^(2))=z, with
0<=z<=R, oriented downward. (a) Parameterize the cone using cylindrical coordinates (write
\theta as theta).
x(r,\theta )=
y(r,\theta )=?
z(r,\theta )=
with <=r<=
and _(()
)<=\theta <=(b) With this parameterization, what is
dvec(A)?
dvec(A)=(c) Find the flux of
vec(F)through
S. flux
=