(Solved): Compute the directional derivative at \( P \) in the direction of \( \mathbf{v} \). \[ f(x, y)=\si ...

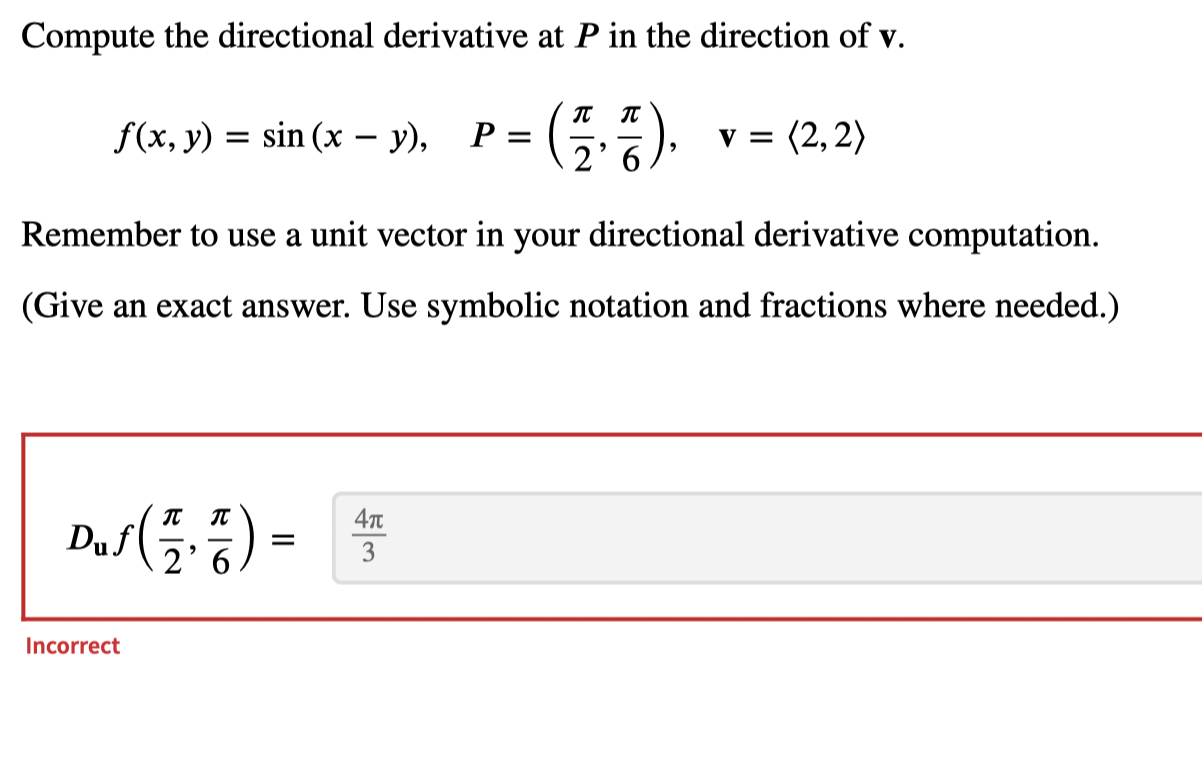
Compute the directional derivative at \( P \) in the direction of \( \mathbf{v} \). \[ f(x, y)=\sin (x-y), \quad P=\left(\frac{\pi}{2}, \frac{\pi}{6}\right), \quad \mathbf{v}=\langle 2,2\rangle \] Remember to use a unit vector in your directional derivative computation. (Give an exact answer. Use symbolic notation and fractions where needed.) \[ D_{\mathbf{u}} f\left(\frac{\pi}{2}, \frac{\pi}{6}\right)= \] Incorrect Compute the directional derivative in the direction of \( \mathbf{v} \) at the given point. \[ f(x, y)=x y^{3}-x^{2}, \quad \mathbf{v}=\mathbf{i}-\mathbf{j}, \quad P=(-1,2) \] Remember to use a unit vector in your directional derivative computation. (Give an exact answer. Use symbolic notation and fractions where needed.) \[ D_{\mathbf{u}} f(-1,2)==\frac{326}{\sqrt{2}} \] Incorrect