(Solved): Can someone help me figure out how to set up a design and observation matrix for the following polar ...

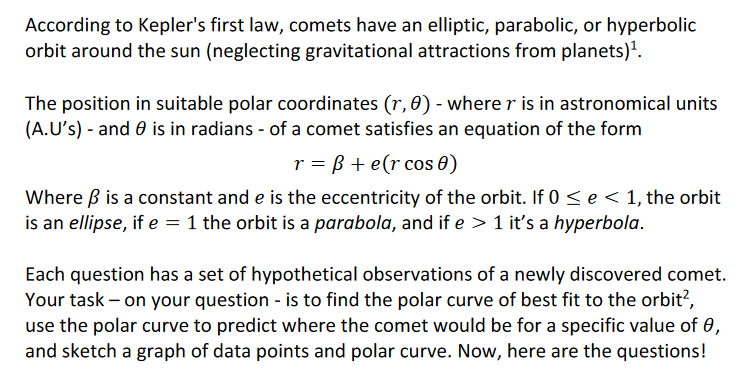
According to Kepler's first law, comets have an elliptic, parabolic, or hyperbolic orbit around the sun (neglecting gravitational attractions from planets) ${}^1$. The position in suitable polar coordinates $(r,?)$ - where $r$ is in astronomical units (A.U's) - and $?$ is in radians - of a comet satisfies an equation of the form $r=?+e(r\cos ?)$ Where $?$ is a constant and $e$ is the eccentricity of the orbit. If $0?e<1$, the orbit is an ellipse, if $e=1$ the orbit is a parabola, and if $e>1$ it's a hyperbola. Each question has a set of hypothetical observations of a newly discovered comet. Your task - on your question - is to find the polar curve of best fit to the orbit ${}^2$, use the polar curve to predict where the comet would be for a specific value of $?$, and sketch a graph of data points and polar curve. Now, here are the questions! 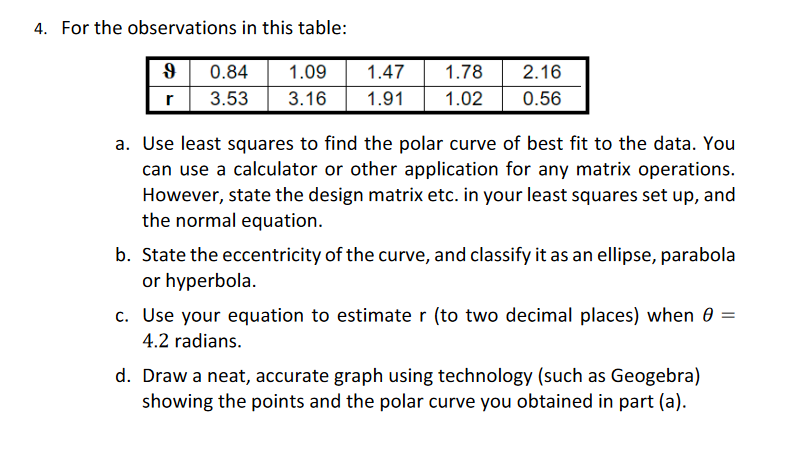4. For the observations in this table: a. Use least squares to find the polar curve of best fit to the data. You can use a calculator or other application for any matrix operations. However, state the design matrix etc. in your least squares set up, and the normal equation. b. State the eccentricity of the curve, and classify it as an ellipse, parabola or hyperbola. c. Use your equation to estimate $r$ (to two decimal places) when $?=$ 4.2 radians. d. Draw a neat, accurate graph using technology (such as Geogebra) showing the points and the polar curve you obtained in part (a).