(Solved): can someone explain the solution in details thx Verifying an Identity Using Algebra and Even/Odd Ide ...

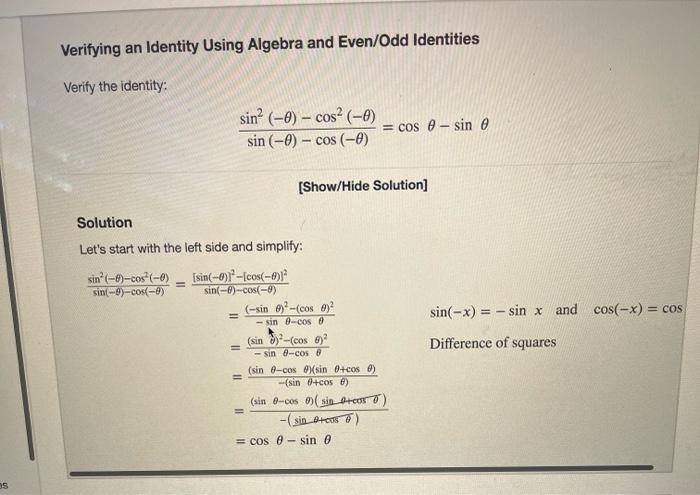
Verifying an Identity Using Algebra and Even/Odd Identities Verify the identity: \[ \frac{\sin ^{2}(-\theta)-\cos ^{2}(-\theta)}{\sin (-\theta)-\cos (-\theta)}=\cos \theta-\sin \theta \] [Show/Hide Solution] Solution Let's start with the left side and simplify: \[ \begin{aligned} \frac{\sin ^{2}(-\theta)-\cos ^{2}(-\theta)}{\sin (-\theta)-\cos (-\theta)}=\frac{[\sin (-\theta))^{2}-[\cos (-\theta)]^{2}}{\sin (-\theta)-\cos (-\theta)} \\ &=\frac{(-\sin \theta)^{2}-(\cos \theta)^{2}}{-\sin \theta-\cos \theta} \\ &=\frac{(\sin \theta)^{2}-(\cos \theta)^{2}}{-\sin \theta-\cos \theta(\sin \theta+\cos \theta)} \\ &=\frac{(\sin \theta-\cos \theta)(-x)=-\sin x \text { and } \cos (-x)=\cos }{-(\sin \theta+\cos \theta)} \\ &=\frac{(\sin \theta-\cos \theta(\sin \theta-\cos \theta)}{-(\sin \theta+\cos \theta)} \\ & \text { Difference of squares } \\ &=\cos \theta-\sin \theta \end{aligned} \]