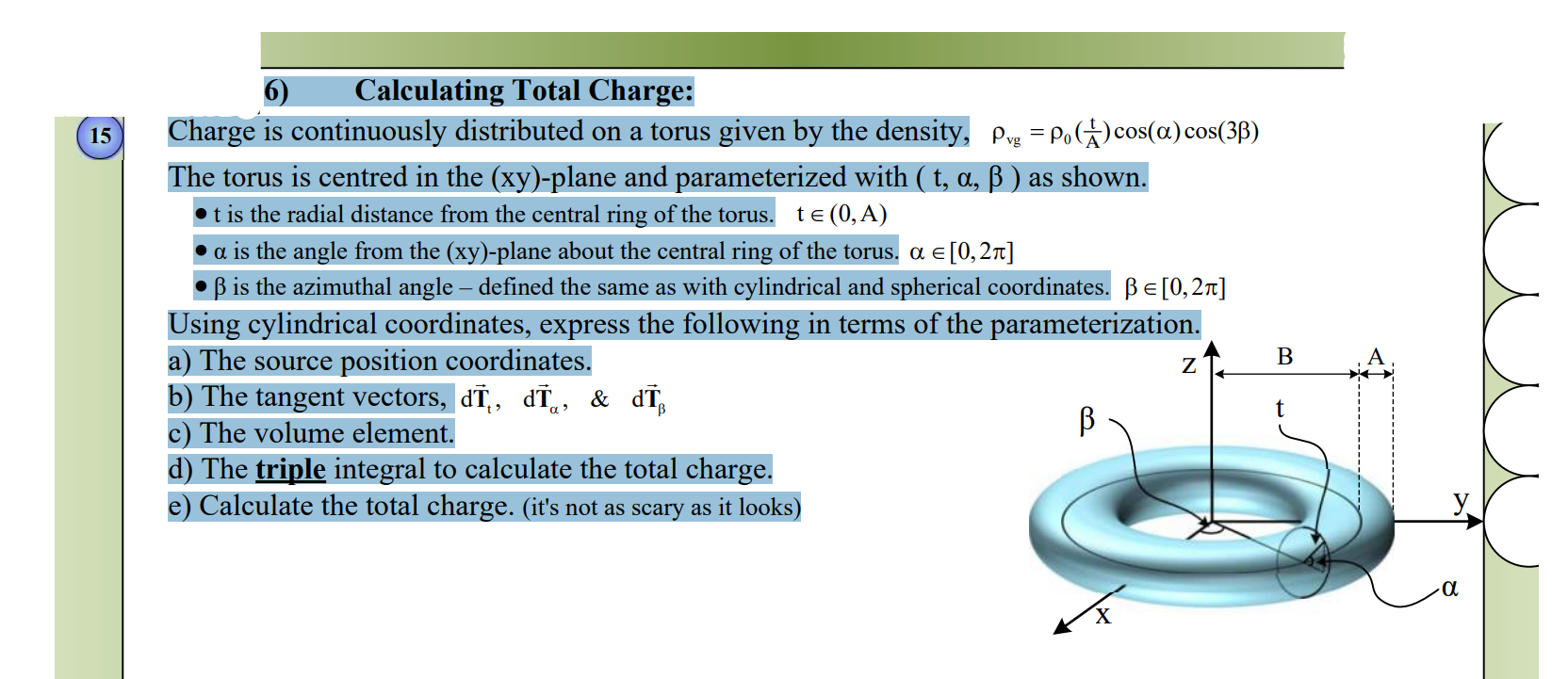
(Solved): Calculating Total Charge: Charge is continuously distributed on a torus given by the density, rho_(v ...
Calculating Total Charge: Charge is continuously distributed on a torus given by the density, rho_(vg)=rho_(0)((t)/((A)))cos(alpha)cos(3beta) The torus is centred in the (xy)-plane and parameterized with (t,alpha,beta) as shown. t is the radial distance from the central ring of the torus. t in(0,A) alpha is the angle from the (xy)-plane about the central ring of the torus. alpha in[0,2pi] beta is the azimuthal angle - defined the same as with cylindrical and spherical coordinates. beta in[0,2pi] Using cylindrical coordinates, express the following in terms of the parameterization. a) The source position coordinates. b) The tangent vectors, d vec(T)_(1),d vec(T)_(alpha),&d vec(T)_(beta) c) The volume element. d) The triple integral to calculate the total