(Solved): BELOW IS THE EQUATIN (2) 3.1 Following the discussion in section 7.2 of the textbook, write down th ...

3.1 Following the discussion in section 7.2 of the textbook, write down the differential equation of the series R-C circuit in the absence of any forcing input. Then, explain or derive equation (1) in your own way. 3.2 For $R=10\mathrm{M}?$ and $C=15?\mathrm{F}$, determine the expected time constant $?=RC$. 3.3 Equation (2) for $V_{\mathrm{CPP}}$ is rather difficult to prove at this time. Take it as a challenge to derive it as you learn increasingly more on the topic of differential equations. 3.4 Explain in your own words why an R-C series circuit can act approximately as an integrator as well as a differentiator and under what conditions. 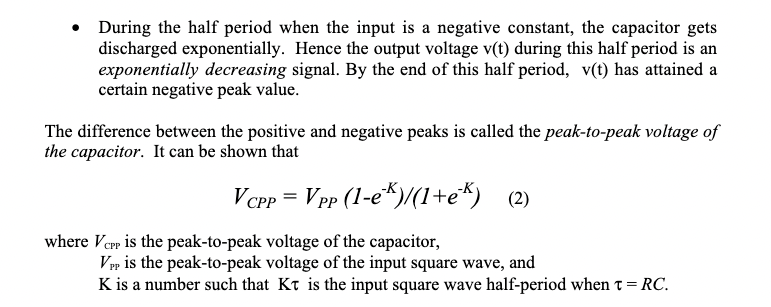- During the half period when the input is a negative constant, the capacitor gets discharged exponentially. Hence the output voltage $v(t)$ during this half period is an exponentially decreasing signal. By the end of this half period, $v(t)$ has attained a certain negative peak value. The difference between the positive and negative peaks is called the peak-to-peak voltage of the capacitor. It can be shown that $V_{CPP}=V_{PP}\left(1?e^{?K}\right)/\left(1+e^{?K}\right)$ where $V_{\mathrm{CPP}}$ is the peak-to-peak voltage of the capacitor, $V_{\mathrm{Pp}}$ is the peak-to-peak voltage of the input square wave, and $\mathrm{K}$ is a number such that $\mathrm{K}?$ is the input square wave half-period when $?=RC$.