(Solved): Basis change to find eigenvalues: Consider an \( N \)-dimensional Hilbert space with orthonormal b ...

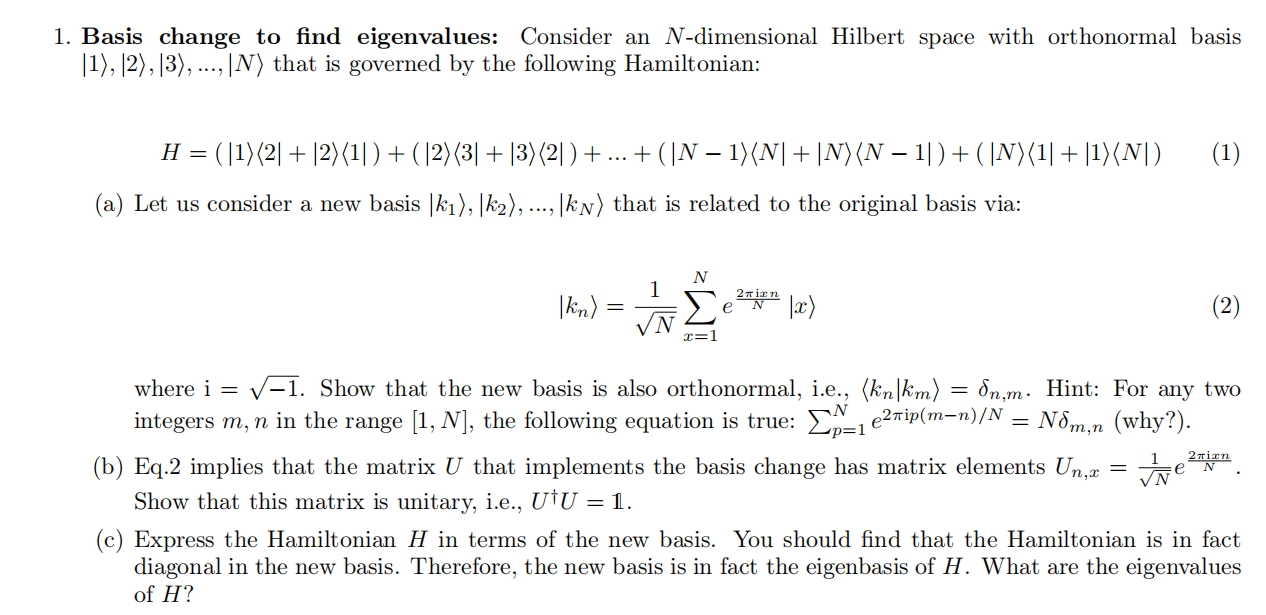
Basis change to find eigenvalues: Consider an \( N \)-dimensional Hilbert space with orthonormal basis \( |1\rangle,|2\rangle,|3\rangle, \ldots,|N\rangle \) that is governed by the following Hamiltonian: \[ H=(|1\rangle\langle 2|+| 2\rangle\langle 1|)+(|2\rangle\langle 3|+| 3\rangle\langle 2|)+\ldots+(|N-1\rangle\langle N|+| N\rangle\langle N-1|)+(|N\rangle\langle 1|+| 1\rangle\langle N|) \] (a) Let us consider a new basis \( \left|k_{1}\right\rangle,\left|k_{2}\right\rangle, \ldots,\left|k_{N}\right\rangle \) that is related to the original basis via: \[ \left|k_{n}\right\rangle=\frac{1}{\sqrt{N}} \sum_{x=1}^{N} e^{\frac{2 \pi \mathrm{i} x n}{N}}|x\rangle \] where \( \mathrm{i}=\sqrt{-1} \). Show that the new basis is also orthonormal, i.e., \( \left\langle k_{n} \mid k_{m}\right\rangle=\delta_{n, m} \). Hint: For any two integers \( m, n \) in the range \( [1, N] \), the following equation is true: \( \sum_{p=1}^{N} e^{2 \pi \mathrm{i} p(m-n) / N}=N \delta_{m, n}( \) why?). (b) Eq.2 implies that the matrix \( U \) that implements the basis change has matrix elements \( U_{n, x}=\frac{1}{\sqrt{N}} e^{\frac{2 \pi \mathrm{i} x n}{N}} \). Show that this matrix is unitary, i.e., \( U^{\dagger} U=\mathbb{1} \). (c) Express the Hamiltonian \( H \) in terms of the new basis. You should find that the Hamiltonian is in fact diagonal in the new basis. Therefore, the new basis is in fact the eigenbasis of \( H \). What are the eigenvalues of \( H \) ?