(Solved): At the time t=0 the wave function for hydrogen atom is (r,0)=101(2100+210+22 ...

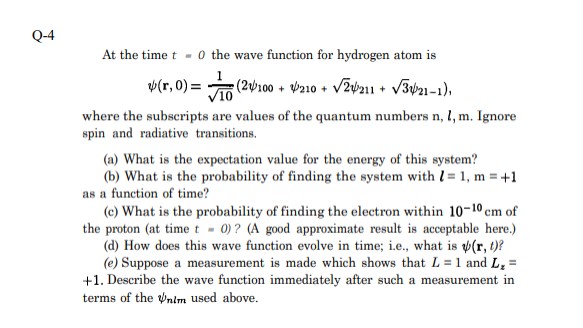
At the time $t=0$ the wave function for hydrogen atom is $?(\mathbf{r},0)=\frac{1}{\sqrt{10}}\left(2{?}_{100}+{?}_{210}+\sqrt{2}{?}_{211}+\sqrt{3}{?}_{21?1}\right),$ where the subscripts are values of the quantum numbers $\mathrm{n},l,\mathrm{m}$. Ignore spin and radiative transitions. (a) What is the expectation value for the energy of this system? (b) What is the probability of finding the system with $l=1,\mathrm{m}=+1$ as a function of time? (c) What is the probability of finding the electron within $10^{?10}\mathrm{cm}$ of the proton (at time $t=0$ ) ? (A good approximate result is acceptable here.) (d) How does this wave function evolve in time; i.e., what is $?(\mathbf{r},t)$ ? (e) Suppose a measurement is made which shows that $L=1$ and $L_z=$ +1 . Describe the wave function immediately after such a measurement in terms of the ${?}_{nlm}$ used above.