(Solved): Assume that an ergodic random process \( X(t, s) \) is unknown but its autoco ...

???????

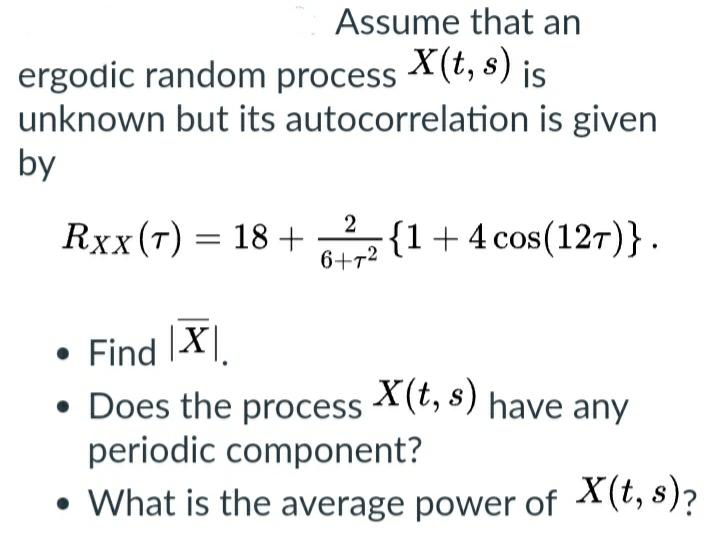
Assume that an ergodic random process \( X(t, s) \) is unknown but its autocorrelation is given by \[ R_{X X}(\tau)=18+\frac{2}{6+\tau^{2}}\{1+4 \cos (12 \tau)\} . \] - Find \( |\bar{X}| \). - Does the process \( X(t, s) \) have any periodic component? - What is the average power of \( X(t, s) \) ?