(Solved): As shown in Figure 4, a uniform thin rod of mass m1, moment of inertia about O of IOzz, a ...

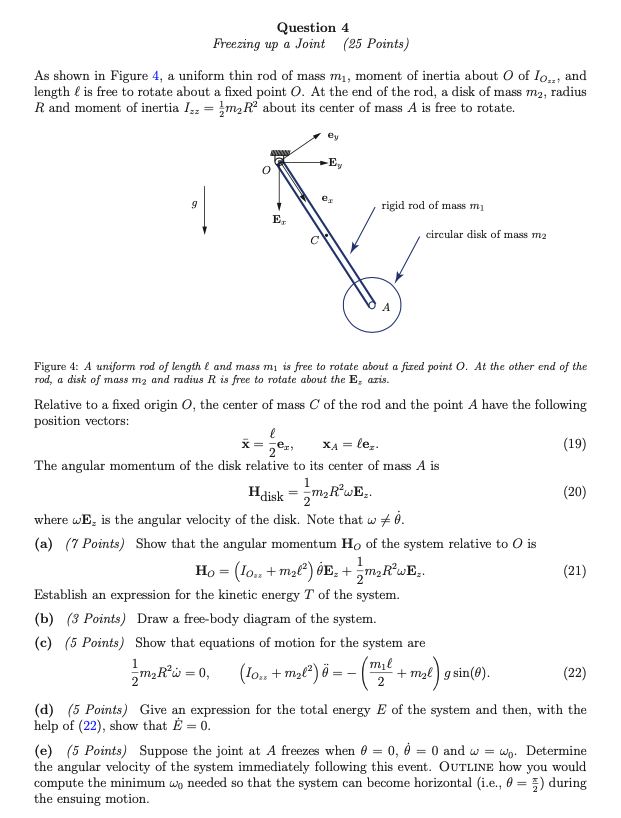
As shown in Figure 4, a uniform thin rod of mass $$m_1$$, moment of inertia about $$O$$ of $$I_{O_{zz}}$$, and length $$?$$ is free to rotate about a fixed point $$O$$. At the end of the rod, a disk of mass $$m_2$$, radius $$R$$ and moment of inertia $$I_{zz}=\frac{1}{2}{m}_2{R}^2$$ about its center of mass $$A$$ is free to rotate. Figure 4: A uniform rod of length $$?$$ and mass $$m_1$$ is free to rotate about a fized point $$O$$. At the other end of the rod, a disk of mass $$m_2$$ and radius $$R$$ is free to rotate about the $${\mathbf{E}}_z$$ aris. Relative to a fixed origin $$O$$, the center of mass $$C$$ of the rod and the point $$A$$ have the following position vectors: $$\overline{\mathbf{x}}=\frac{?}{2}{\mathbf{e}}_x,{\mathbf{x}}_A=?{\mathbf{e}}_x.$$ The angular momentum of the disk relative to its center of mass $$A$$ is $${\mathbf{H}}_{\mathrm{disk}}=\frac{1}{2}{m}_2{R}^2?{\mathbf{E}}_z\text{. }$$ where $$?{\mathbf{E}}_z$$ is the angular velocity of the disk. Note that $$??=\stackrel{?}{?}$$. (a) (7 Points) Show that the angular momentum $${\mathbf{H}}_O$$ of the system relative to $$O$$ is $${\mathbf{H}}_O=\left(I_{O_{az}}+m_2{?}^2\right)\stackrel{?}{?}{\mathbf{E}}_z+\frac{1}{2}{m}_2{R}^2?{\mathbf{E}}_z.$$ Establish an expression for the kinetic energy $$T$$ of the system. (b) (3 Points) Draw a free-body diagram of the system. (c) (5 Points) Show that equations of motion for the system are $$\frac{1}{2}{m}_2{R}^2\stackrel{?}{?}=0,\left(I_{O_{zB}}+m_2{?}^2\right)\ddot{?}=?\left(\frac{m_1?}{2}+m_2?\right)g\sin (?).$$ (d) (5 Points) Give an expression for the total energy $$E$$ of the system and then, with the help of $$(22)$$, show that $$\stackrel{?}{E}=0$$. (e) (5 Points) Suppose the joint at $$A$$ freezes when $$?=0,\stackrel{?}{?}=0$$ and $$?={?}_0$$. Determine the angular velocity of the system immediately following this event. OutLine how you would compute the minimum $${?}_0$$ needed so that the system can become horizontal (i.e., $$?=\frac{?}{2}$$ ) during the ensuing motion.