(Solved): Archimedes supposedly was asked to determine whether a crown made for the king consisted of pure ...

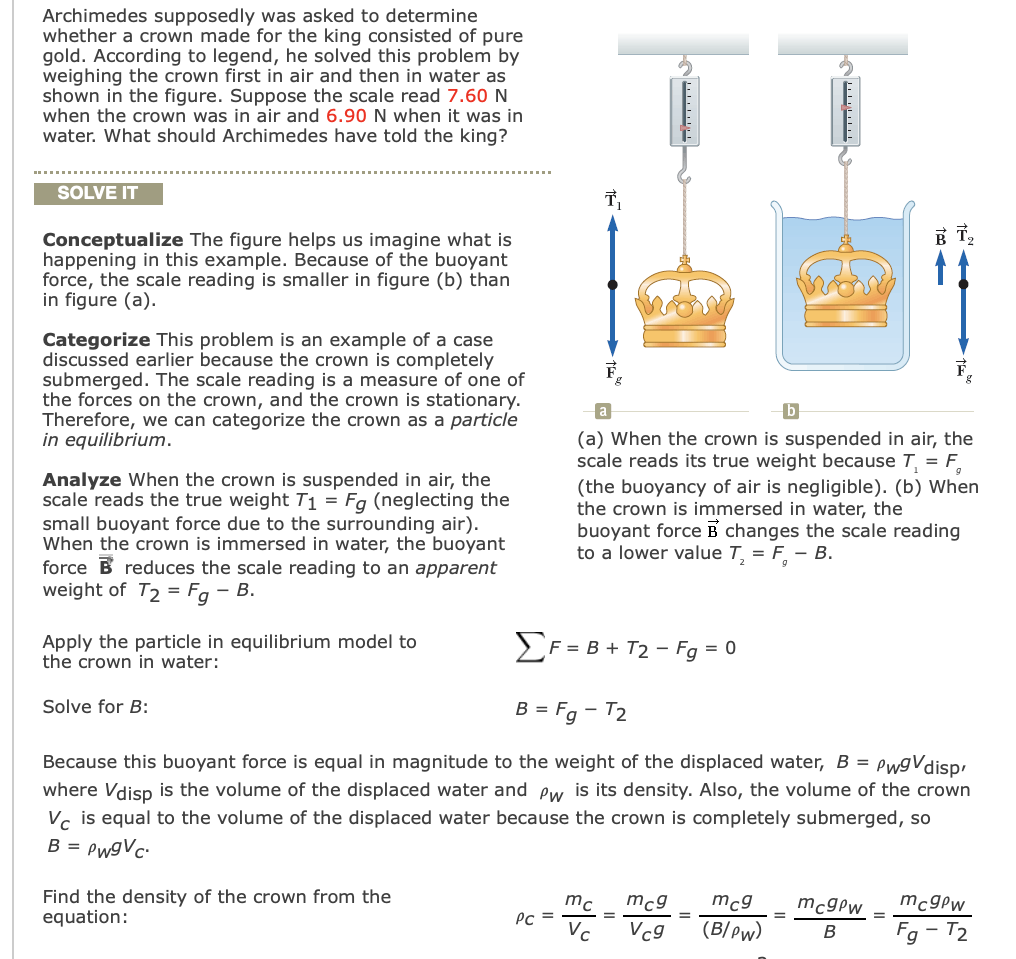
Archimedes supposedly was asked to determine whether a crown made for the king consisted of pure gold. According to legend, he solved this problem by weighing the crown first in air and then in water as shown in the figure. Suppose the scale read \( 7.60 \mathrm{~N} \) when the crown was in air and \( 6.90 \mathrm{~N} \) when it was in water. What should Archimedes have told the king? Conceptualize The figure helps us imagine what is happening in this example. Because of the buoyant force, the scale reading is smaller in figure (b) than in figure (a). Categorize This problem is an example of a case discussed earlier because the crown is completely submerged. The scale reading is a measure of one of the forces on the crown, and the crown is stationary. Therefore, we can categorize the crown as a particle in equilibrium. (a) When the crown is suspended in air, the Analyze When the crown is suspended in air, the scale reads its true weight because \( T_{1}=F_{g} \) scale reads the true weight \( T_{1}=F_{g} \) (neglecting the \( \quad \) (the buoyancy of air is negligible). (b) When small buoyant force due to the surrounding air). the crown is immersed in water, the When the crown is immersed in water, the buoyant buoyant force \( \overrightarrow{\mathbf{B}} \) changes the scale reading force \( \overline{\bar{B}} \) reduces the scale reading to an apparent to a lower value \( T_{2}=F_{g}-B \). weight of \( T_{2}=F_{g}-B \). \( \begin{array}{l}\text { Apply the particle in equilibrium model to } \\ \text { the crown in water: }\end{array} \quad \quad \quad F=B+T_{2}-F_{g}=0 \) Solve for \( B \) : \[ B=F_{g}-T_{2} \] Because this buoyant force is equal in magnitude to the weight of the displaced water, \( B=\rho_{W} g V_{\mathrm{disp}} \) where \( V_{\text {disp }} \) is the volume of the displaced water and \( \rho_{W} \) is its density. Also, the volume of the crown \( V_{C} \) is equal to the volume of the displaced water because the crown is completely submerged, so \[ B=\rho_{w} g V_{C} \text {. } \] Find the density of the crown from the equation: \[ \rho_{C}=\frac{m_{C}}{V_{C}}=\frac{m_{C} g}{V_{C} g}=\frac{m_{C} g}{\left(B / \rho_{W}\right)}=\frac{m_{C} g \rho_{W}}{B}=\frac{m_{C} g \rho_{W}}{F_{g}-T_{2}} \] Find the density of the crown from the equation: \[ \begin{aligned} \rho_{C} & =\frac{m_{C}}{V_{C}}=\frac{m_{C} g}{V_{C} g}=\frac{m_{C} g}{\left(B / \rho_{W}\right)}=\frac{m_{C} g \rho_{W}}{B}=\frac{m_{C} g \rho_{W}}{F_{g}-T_{2}} \\ \rho_{C} & =\frac{(7.60 \mathrm{~N})\left(1,000 \mathrm{~kg} / \mathrm{m}^{3}\right)}{7.60 \mathrm{~N}-6.90 \mathrm{~N}} \end{aligned} \] Substitute numerical values: \[ \rho_{C}=10857.14286 \backsim \mathrm{kg} / \mathrm{m}^{3} \] Finalize From this table, we see that the density of gold is \( 19.3 \times 10^{3} \mathrm{~kg} / \mathrm{m}^{3} \). Therefore, Archimedes should have reported that the king had been cheated. Either the crown was hollow, or it was not made of pure gold. HINTS: (a) In a similar experiment, if we want to buoy a \( 0.157-\mathrm{kg} \) solid platinum object in water with an attached strinq from above, what is the tension required to support the piece of platinum? Use Archimedes's principle and the tabulated density of platinum to find the buoyant force. \( \mathrm{N} \) (b) If the object is now attached to a piece of Styrofoam, what volume of Styrofoam (density \( \rho_{S}=74 \mathrm{~kg} / \mathrm{m}^{3} \) ) should be used to have the Styrofoam and the metal floating just under the water surface? Construct an expression for the overall density of the combined platinum and Styrofoam, and use Archimedes's principle to impose the condition that it just barely floats. \( \mathrm{m}^{3} \)