Home /
Expert Answers /
Electrical Engineering /
an-infinite-horizontal-wire-carries-a-current-i-linear-density-of-charge-moving-at-a-velocity-pa139
(Solved): An infinite horizontal wire carries a current I (linear density of charge , moving at a velocity ...
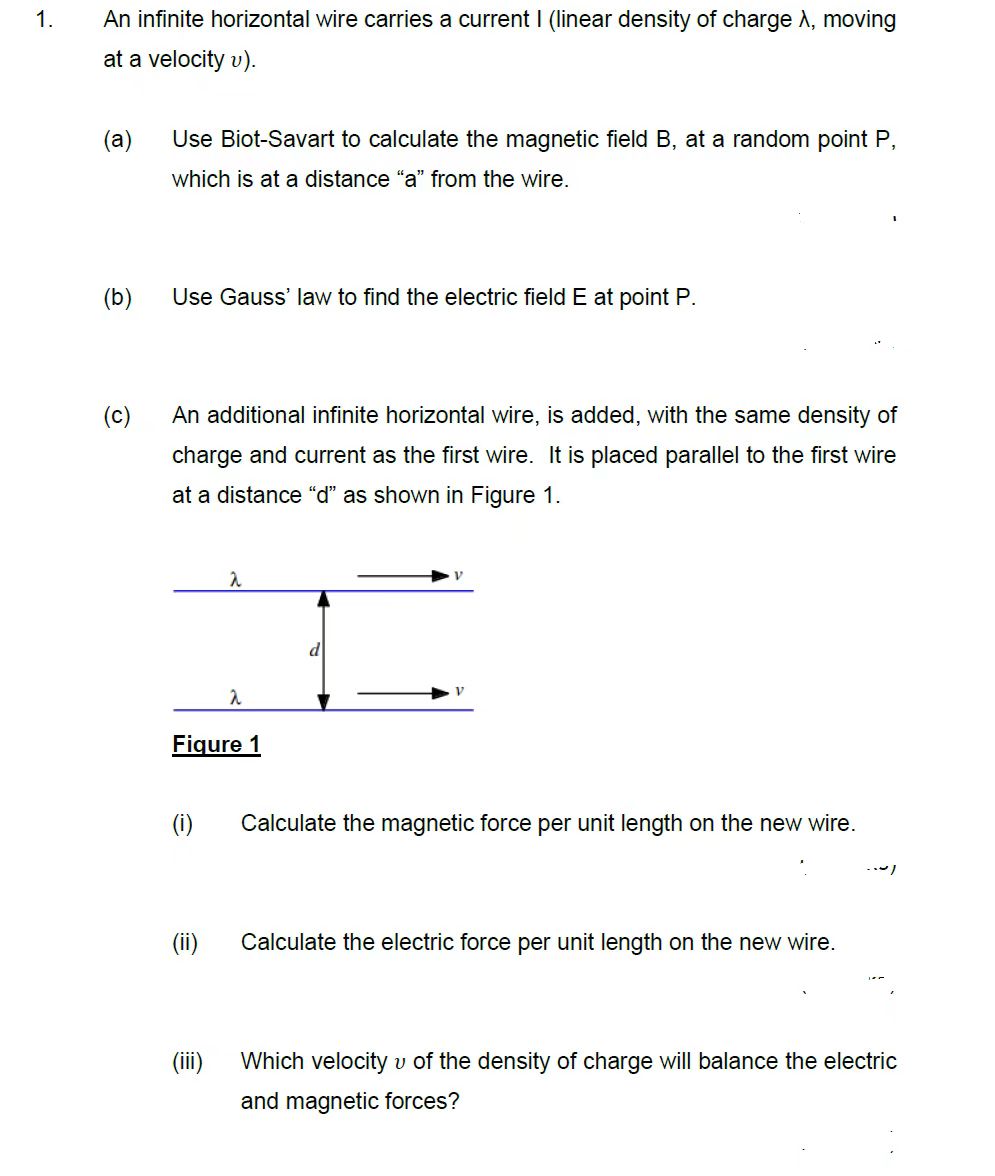
An infinite horizontal wire carries a current I (linear density of charge , moving at a velocity ). (a) Use Biot-Savart to calculate the magnetic field , at a random point , which is at a distance "a" from the wire. (b) Use Gauss' law to find the electric field at point . (c) An additional infinite horizontal wire, is added, with the same density of charge and current as the first wire. It is placed parallel to the first wire at a distance "d" as shown in Figure 1. Fiqure 1 (i) Calculate the magnetic force per unit length on the new wire. (ii) Calculate the electric force per unit length on the new wire. (iii) Which velocity of the density of charge will balance the electric and magnetic forces?
Expert Answer
(a) The magnetic field at point P can be calculated using the Biot-Savart Law by:dB = ?0/4? * Idl x r / r^3where Idl is the current element, r is the separation between Idl and point P, x is the cross product, and 0 is the permeability of empty space.We can assume that the current element Idl is equal to dl because the wire is infinite and uniformly charged with linear density of charge, where ?dl is an element of length along the wire. The current flow, which is perpendicular to the wire and into the page, determines the direction of Idl.Consider a wire element of length dl that is located x distance away from point P. r^2 = a^2 + x^2 calculates the distance r between the current element and point P. The right-hand rule specifies the direction of dB, which is perpendicular to both Idl and r.As a result, integrating throughout the wire's length yields the total magnetic field at point P due to the entire wire:B = ?dB = ?0/4? * ??vdl x r / r^3= ?0/4? * ?v ?(dx/sqrt(a^2+x^2))(i - j)= ?0?v/4? * ln[(a + ?(a^2+d^2))/(a - ?(a^2+d^2))] * iwhere i is the unit vector pointing in the direction of the reader and perpendicular to the plane that contains the wire and point P.The Biot-Savart Law can be used to determine the magnetic field at a point P as a result of a wire carrying electricity. According to this law, the current element Idl, the distance r between it and point P, and the angle formed by the two are all proportional to the magnetic field at point P. The total magnetic field at point P is obtained by integrating the magnetic field along the length of the wire. According to the right-hand rule, the magnetic field's direction is perpendicular to the current element and the distance between it and point P. If the current element Idl equals dl, the wire is infinite and uniformly charged with linear density of charge, and the current flows perpendicular to the wire and into the page, the equation for the magnetic field at point P can be made simpler.