(Solved): According to the calculations mentioned below, draw the shear and bending moment diagram of all beam ...

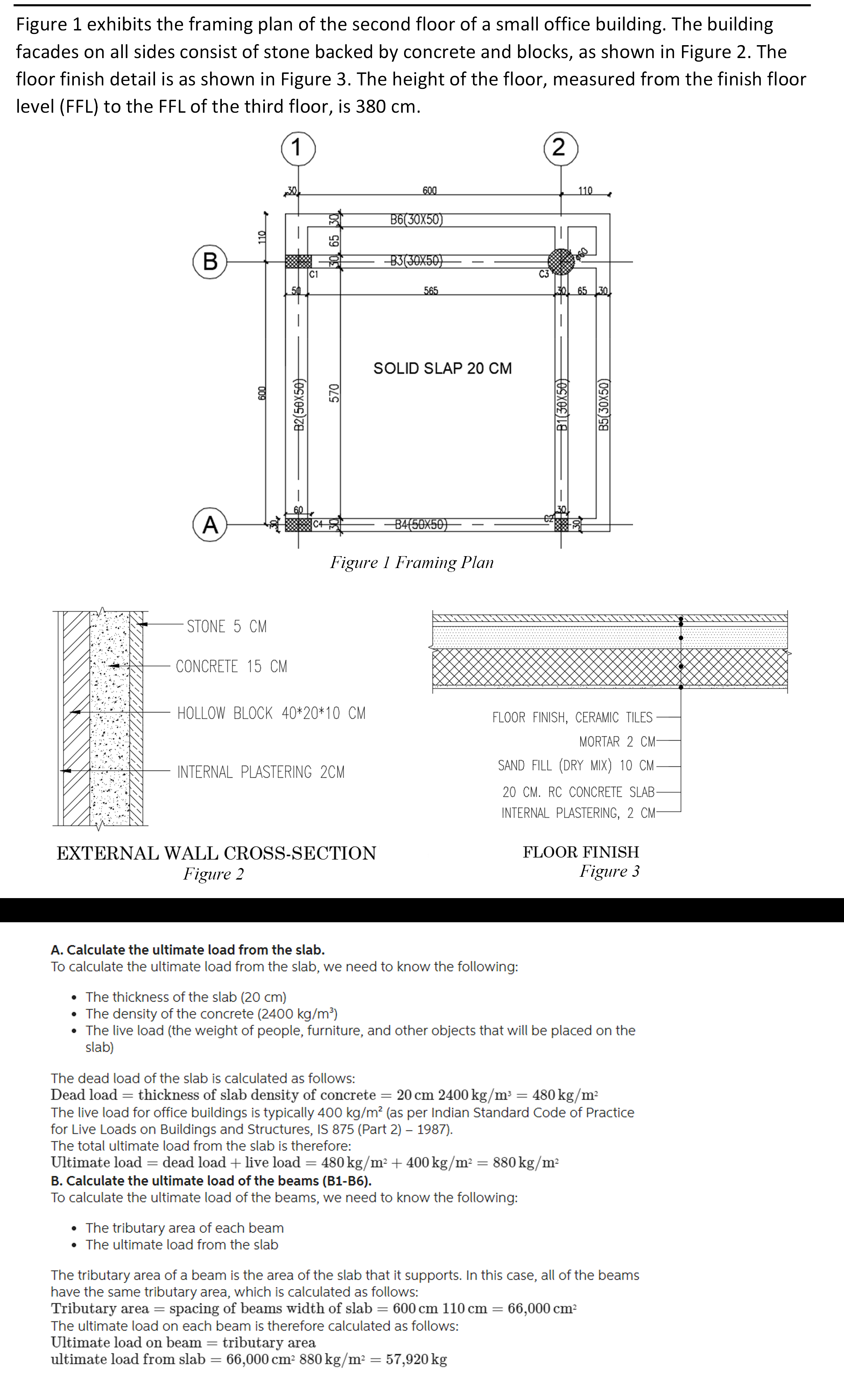
C. Draw the shear and bending moment diagrams of all beams using the ultimate loads. Figure 1 exhibits the framing plan of the second floor of a small office building. The building facades on all sides consist of stone backed by concrete and blocks, as shown in Figure 2 . The floor finish detail is as shown in Figure 3. The height of the floor, measured from the finish floor level (FFL) to the FFL of the third floor, is $380\mathrm{cm}$. EXTERNAL WALL CROSS-SECTION Figure 2 FLOOR FINISH Figure 3 A. Calculate the ultimate load from the slab. To calculate the ultimate load from the slab, we need to know the following: - The thickness of the slab $(20\mathrm{cm})$ - The density of the concrete $\left(2400\mathrm{kg}/{\mathrm{m}}^3\right)$ - The live load (the weight of people, furniture, and other objects that will be placed on the slab) The dead load of the slab is calculated as follows: Dead load $=$ thickness of slab density of concrete $=20\mathrm{cm}2400\mathrm{kg}/{\mathrm{m}}^3=480\mathrm{kg}/{\mathrm{m}}^2$ The live load for office buildings is typically $400\mathrm{kg}/{\mathrm{m}}^2$ (as per Indian Standard Code of Practice for Live Loads on Buildings and Structures, IS 875 (Part 2) - 1987). The total ultimate load from the slab is therefore: Ultimate load $=$ dead load + live load $=480\mathrm{kg}/{\mathrm{m}}^2+400\mathrm{kg}/{\mathrm{m}}^2=880\mathrm{kg}/{\mathrm{m}}^2$ B. Calculate the ultimate load of the beams (B1-B6). To calculate the ultimate load of the beams, we need to know the following: - The tributary area of each beam - The ultimate load from the slab The tributary area of a beam is the area of the slab that it supports. In this case, all of the beams have the same tributary area, which is calculated as follows: Tributary area $=$ spacing of beams width of slab $=600\mathrm{cm}110\mathrm{cm}=66,000{\mathrm{cm}}^2$ The ultimate load on each beam is therefore calculated as follows: Ultimate load on beam $=$ tributary area ultimate load from slab $=66,000{\mathrm{cm}}^{2}880\mathrm{kg}/{\mathrm{m}}^2=57,920\mathrm{kg}$