(Solved): A5. A two-dimensional, incompressible, irrotational fluid flow is given by the complex potential \ ...

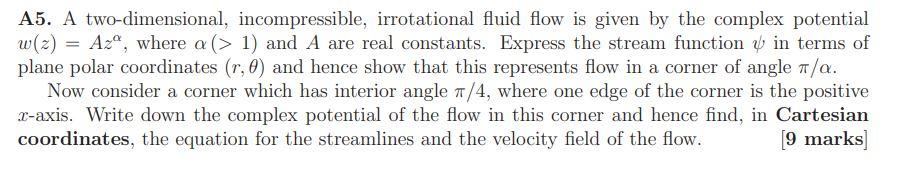
A5. A two-dimensional, incompressible, irrotational fluid flow is given by the complex potential \( w(z)=A z^{\alpha} \), where \( \alpha(>1) \) and \( A \) are real constants. Express the stream function \( \psi \) in terms of plane polar coordinates \( (r, \theta) \) and hence show that this represents flow in a corner of angle \( \pi / \alpha \). Now consider a corner which has interior angle \( \pi / 4 \), where one edge of the corner is the positive \( x \)-axis. Write down the complex potential of the flow in this corner and hence find, in Cartesian coordinates, the equation for the streamlines and the velocity field of the flow. \( [9 \) marks \( ] \)