(Solved): A tubular column of length \( l \) is fixed at the base and free at the top, supporting a load \( ...

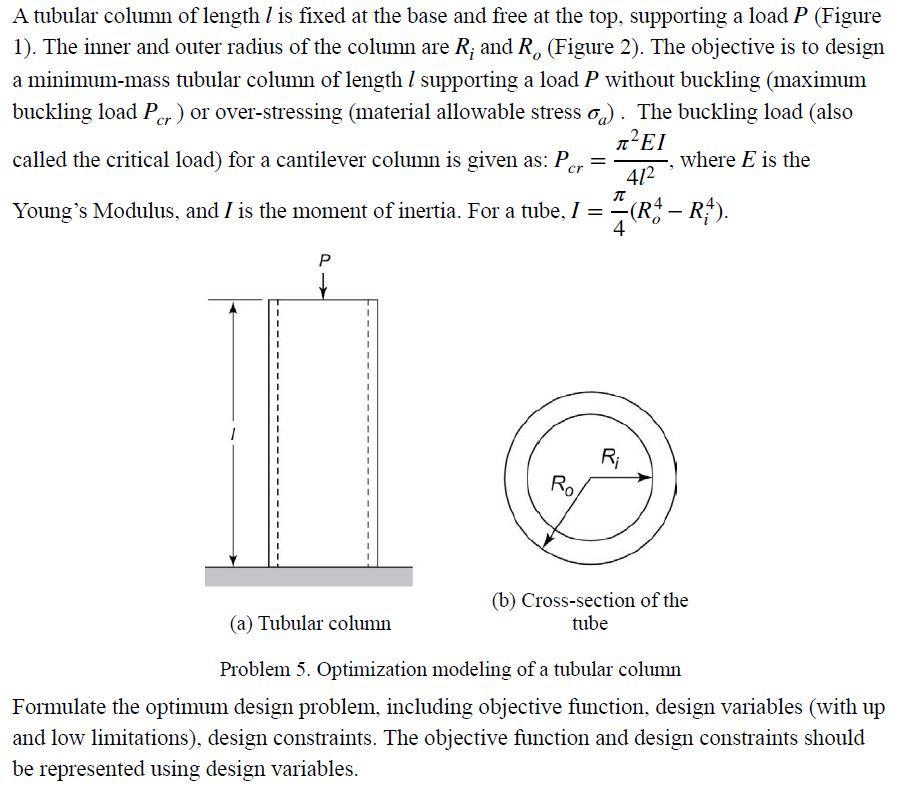
A tubular column of length \( l \) is fixed at the base and free at the top, supporting a load \( P \) (Figure 1). The inner and outer radius of the column are \( R_{i} \) and \( R_{o} \) (Figure 2). The objective is to design a minimum-mass tubular column of length \( l \) supporting a load \( P \) without buckling (maximum buckling load \( P_{c r} \) ) or over-stressing (material allowable stress \( \sigma_{a} \) ). The buckling load (also called the critical load) for a cantilever column is given as: \( P_{c r}=\frac{\pi^{2} E I}{4 l^{2}} \), where \( E \) is the Young's Modulus, and \( I \) is the moment of inertia. For a tube, \( I=\frac{\pi}{4}\left(R_{o}^{4}-R_{i}^{4}\right) \). (a) Tubular column (b) Cross-section of the tube Problem 5. Optimization modeling of a tubular column Formulate the optimum design problem, including objective function, design variables (with up and low limitations), design constraints. The objective function and design constraints should be represented using design variables.