(Solved): (a) Suppose that i 1 is a positive such that Vi = Vi+1. Prove that V() = Vi. (b) In the lectur ...

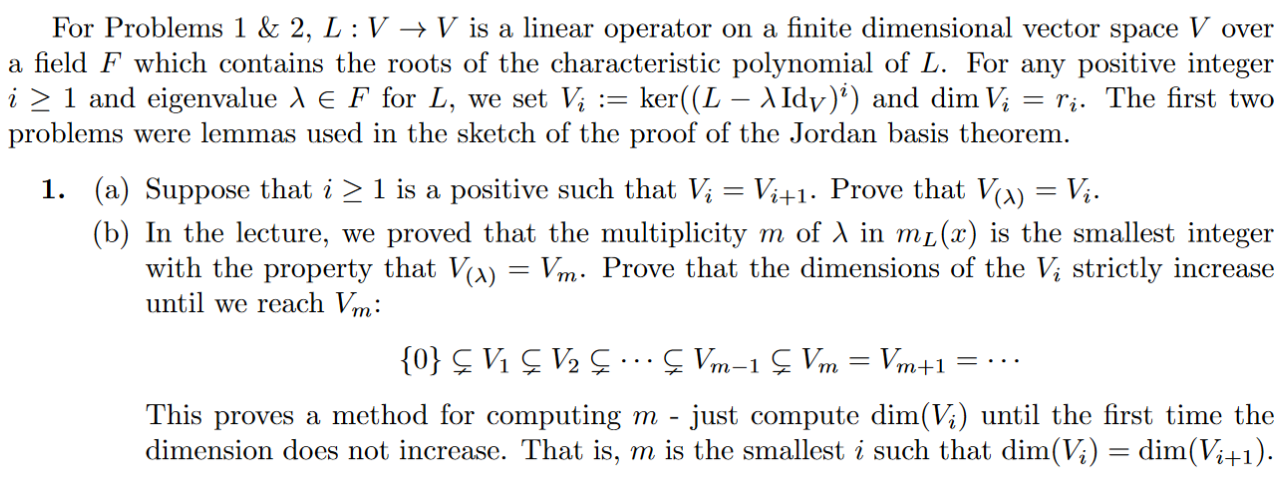
(a) Suppose that i ? 1 is a positive such that Vi = Vi+1. Prove that V(?) = Vi.
(b) In the lecture, we proved that the multiplicity m of ? in mL(x) is the smallest integer
with the property that V(?) = Vm. Prove that the dimensions of the Vi strictly increase
until we reach Vm:
{0} ? V1 ? V2 ? · · · ? Vm?1 ? Vm = Vm+1 = · · ·
This proves a method for computing m - just compute dim(Vi) until the first time the
dimension does not increase. That is, m is the smallest i such that dim(Vi) = dim(Vi+1)

For Problems \( 1 \& 2, L: V \rightarrow V \) is a linear operator on a finite dimensional vector space \( V \) over a field \( F \) which contains the roots of the characteristic polynomial of \( L \). For any positive integer \( i \geq 1 \) and eigenvalue \( \lambda \in F \) for \( L \), we set \( V_{i}:=\operatorname{ker}\left(\left(L-\lambda \operatorname{Id}_{V}\right)^{i}\right) \) and \( \operatorname{dim} V_{i}=r_{i} \). The first two problems were lemmas used in the sketch of the proof of the Jordan basis theorem. 1. (a) Suppose that \( i \geq 1 \) is a positive such that \( V_{i}=V_{i+1} \). Prove that \( V_{(\lambda)}=V_{i} \). (b) In the lecture, we proved that the multiplicity \( m \) of \( \lambda \) in \( m_{L}(x) \) is the smallest integer with the property that \( V_{(\lambda)}=V_{m} \). Prove that the dimensions of the \( V_{i} \) strictly increase until we reach \( V_{m} \) : \[ \{0\} \subsetneq V_{1} \subsetneq V_{2} \subsetneq \cdots \subsetneq V_{m-1} \subsetneq V_{m}=V_{m+1}=\cdots \] This proves a method for computing \( m \) - just compute \( \operatorname{dim}\left(V_{i}\right) \) until the first time the dimension does not increase. That is, \( m \) is the smallest \( i \) such that \( \operatorname{dim}\left(V_{i}\right)=\operatorname{dim}\left(V_{i+1}\right) \).