(Solved): A solid steel bar of diameter \( d_{1}=25 \mathrm{~mm} \) is enclosed by a steel tube of outer diam ...

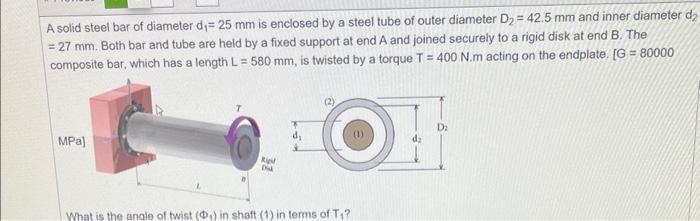
A solid steel bar of diameter \( d_{1}=25 \mathrm{~mm} \) is enclosed by a steel tube of outer diameter \( D_{2}=42.5 \mathrm{~mm} \) and inner diameter \( d_{2} \) \( =27 \mathrm{~mm} \). Both bar and tube are held by a fixed support at end \( A \) and joined securely to a rigid disk at end \( B \). The composite bar, which has a length \( L=580 \mathrm{~mm} \), is twisted by a torque \( T=400 \) N.m acting on the endplate. [G=80000 What is the anale of twist \( \left(\Phi_{1}\right) \) in shaft (1) in terms of \( T_{1} \) ? What is the relationship between internal torques in element (1) and (2) from the compatibility of deformation? \[ \text { 5.56 } T_{1}=T_{2} \] What is the internal torque in shaft (1) \( T_{1} \) ? \[ 55 \mathrm{Nm} \] What is the maximum shear stress in segment (2)? What is the angle of rotation of the endplate?