(Solved): A solid cylindrical needle of diameter \( d \) and length \( L \) is floating on the water surface ...

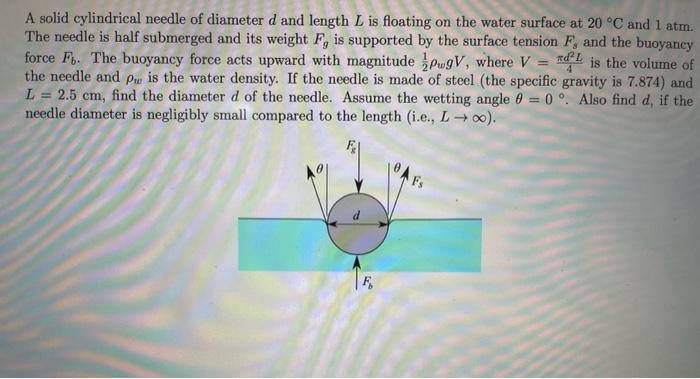
A solid cylindrical needle of diameter \( d \) and length \( L \) is floating on the water surface at \( 20^{\circ} \mathrm{C} \) and \( 1 \mathrm{~atm} \). The needle is half submerged and its weight \( F_{g} \) is supported by the surface tension \( F_{g} \) and the buoyancy force \( F_{b} \). The buoyancy force acts upward with magnitude \( \frac{1}{2} \rho_{w} g V \), where \( V=\frac{\pi d^{2} L}{4} \) is the volume of the needle and \( \rho_{w} \) is the water density. If the needle is made of steel (the specific gravity is 7.874) and \( L=2.5 \mathrm{~cm} \), find the diameter \( d \) of the needle. Assume the wetting angle \( \theta=0{ }^{\circ} \). Also find \( d \), if the needle diameter is negligibly small compared to the length (i.e., \( L \rightarrow \infty \) ).