(Solved): A rain drop hitting a lake makes a circular ripple. Suppose the radius, in inches, grows as a funct ...

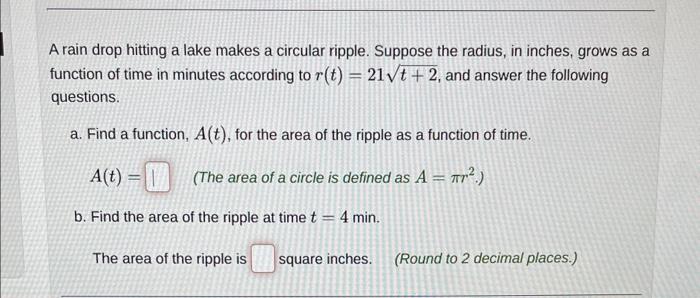
A rain drop hitting a lake makes a circular ripple. Suppose the radius, in inches, grows as a function of time in minutes according to \( r(t)=21 \sqrt{t+2} \), and answer the following questions. a. Find a function, \( A(t) \), for the area of the ripple as a function of time. \( A(t)=\quad \) (The area of a circle is defined as \( A=\pi r^{2} \).) b. Find the area of the ripple at time \( t=4 \mathrm{~min} \). The area of the ripple is square inches. (Round to 2 decimal places.) Use function composition to verify that \( f(x)=(x-5)\left(\frac{1}{3}\right) \) and \( g(x)=x^{3}+5 \) are inverse functions. (You are strongly encouraged to work this question on paper first, then use your written work to fill in the blanks.) a. \( f(g(x))=f(\quad)=(\quad-5)^{\frac{1}{3}}=(\quad)^{\frac{1}{3}}= \) b. \( g(f(x))=g(\quad)=(\quad)^{3}+5=\quad+5= \) Use the function \( f(x)=-3-2 x \) to answer the following. a. \( f^{-1}(x)= \) b. \( \left(f \circ f^{-1}(x)\right)= \) c. \( f^{-1}(f(x))= \) d. Part b. and Part c. verify the result for part a. because the definition of an inverse states the composition of a function and it's inverse is equal to