(Solved): (a) Prove from first principles or using the limit along the curve to show that the following hmit ...

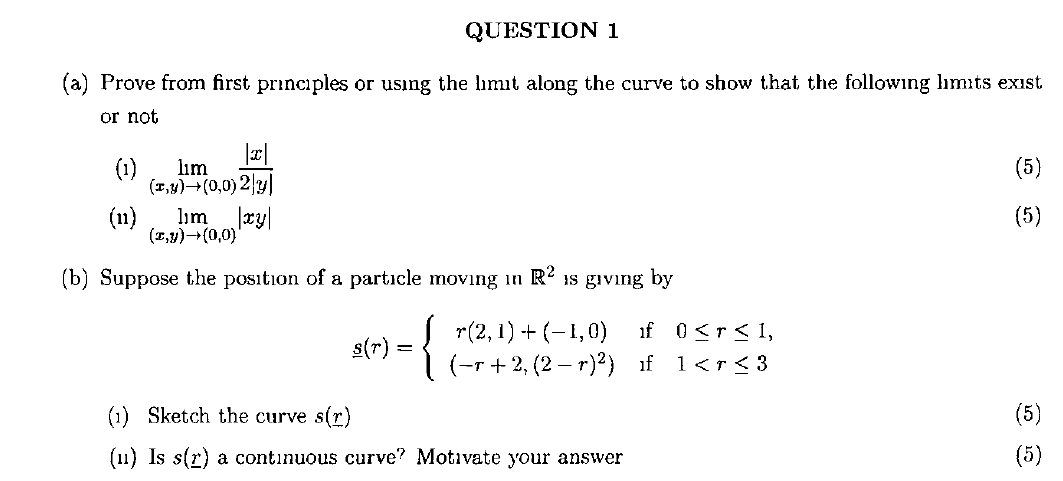
(a) Prove from first principles or using the limit along the curve to show that the following hmits exist or not (1) \( \lim _{(x, y) \rightarrow(0,0)} \frac{|x|}{2|y|} \) (11) \( \lim _{(x, y) \rightarrow(0,0)}|x y| \) (b) Suppose the position of a particle moving in \( \mathbb{R}^{2} \) is glving by \[ \underline{s}(r)=\left\{\begin{array}{ccc} r(2,1)+(-1,0) & \text { if } & 0 \leq r \leq 1 \\ \left(-r+2,(2-r)^{2}\right) & \text { if } & 1