(Solved): A particle that moves along a straight line has velocity \( v(t)=t^{2} e^{-4 t} \mathrm{~m} / \math ...

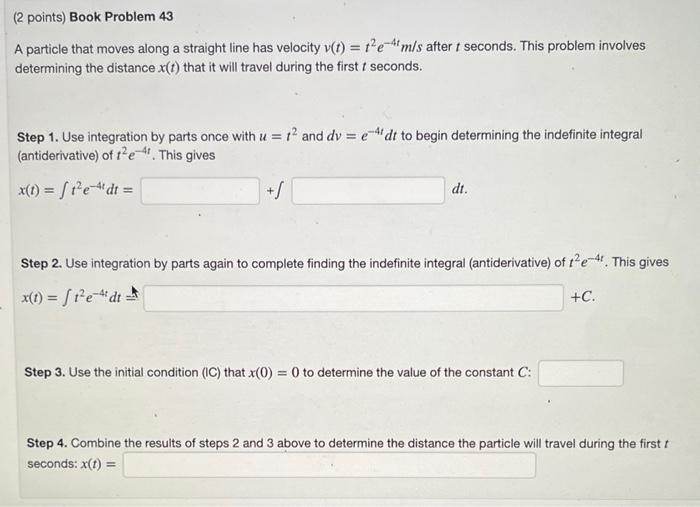
A particle that moves along a straight line has velocity \( v(t)=t^{2} e^{-4 t} \mathrm{~m} / \mathrm{s} \) after \( t \) seconds. This problem involves determining the distance \( x(t) \) that it will travel during the first \( t \) seconds. Step 1. Use integration by parts once with \( u=t^{2} \) and \( d v=e^{-4 t} d t \) to begin determining the indefinite integral (antiderivative) of \( t^{2} e^{-4 t} \). This gives \[ x(t)=\int t^{2} e^{-4 t} d t= \] Step 2. Use integration by parts again to complete finding the indefinite integral (antiderivative) of \( t^{2} e^{-4 t} \). This gives \[ x(t)=\int t^{2} e^{-4 t} a \] Step 3. Use the initial condition (IC) that \( x(0)=0 \) to determine the value of the constant \( C \) :