(Solved): A particle moves in the xy-plane, starting from the origin at \( f=0 \) with an initial velocity ha ...

A particle moves in the xy-plane, starting from the origin at \( f=0 \) with an initial velocity having an \( x \)-component of \( 30 \mathrm{~m} / \mathrm{s} \) and a \( y \) component of \( -10 \mathrm{~m} / \mathrm{s} \). The particle experiences an acceleration in the \( x \)-direction, glven by \( a_{x}=4.5 \mathrm{~m} / \mathrm{s}^{2} \). (a) Determine the total velocity vector at any time. SOt.w110N Conceptualize The components of the initial velocity tell us that the particle starts by moving toward the The x-component of velocity starts at \( 30 \mathrm{~m} / \mathrm{s} \) and increases by \( 4.5 \mathrm{~m} / \mathrm{s} \) every second. The \( y \)-component of velocity never. changes from its initial value of \( -10 \mathrm{~m} / \mathrm{s} \). We sketch a motion diagram of the situation in the figure. Because the particle is accelerating in the +x-direction, its velocity component in this direction increases and the path curves as shown in the diagram. Notice that the spacing between successive images increases as time goes on because the speed is The placement of the acceleration and velocity vectors in the figure helps us further conceptualize the situation. Categorize Because the initial velocity has components in both the \( x \) and \( y \) directions, we categorize this problem as one involving a particle movina in two dimensions, Because the particle only has an x-combonent of acceleratian, we model it as a particle under in the \( x \)-direction and a particle under in the ydirection. Analyze To begin the mathematical analysis, we set \( v_{x e}=30 \mathrm{~m} / \mathrm{s}, v_{y i}=-10 \mathrm{~m} / \mathrm{s}, a_{x}=4.5 \mathrm{~m} / \mathrm{s}^{2} \), and \( a_{y}=0 \mathrm{~m} / \mathrm{s}^{2} \). Use the following equation for the velocity vector: \[ \overrightarrow{\mathrm{v}}_{f}=\overrightarrow{\mathrm{v}}_{i}+\overrightarrow{\mathrm{a}} t=\left(v_{x i}+a_{\gamma} t\right) \hat{i}+\left(v_{y i}+a_{y} t\right) \mathbf{j} \] Substitute numerical values such that \( \vec{v}_{f} \) is in \( \mathrm{m} / \mathrm{s} \) when \( t \) is in \( s \) : (1) \( \vec{v}_{f}=[( \) \( (1) \mathbf{i}+ \) Finalize Notice that the x-component of velocity increases in time whille the \( y \)-component remains constant; this result is consistent with what we predicted. Calculate the velocity and speed of the particle at \( t=8.0 \mathrm{~s} \) and the angle the velocity vector makes with the \( x \)-axis. SOLU110N Analyze Evaluate the result from Equation \( (1) \) at \( t=8.0 \mathrm{~s} \) to solve for \( \vec{v}_{f}(\mathrm{in} \mathrm{m} / \mathrm{s}) \). \( \vec{v}_{f}=[\{\mathbf{i}+[\quad \times \mathrm{j}] \mathrm{m} / \mathrm{s} \) Determine the angle \( \theta \) that \( \vec{v} \) makes with the \( x \) axis at \( t=8.05 \). (Give the smallest-magnitude negative angle below the \( +x \)-axis in degrees.) \[ \theta=\tan ^{-1}\left(\frac{v_{x f}}{v_{x d}}\right)= \] Evaluate the speed of the particle as the magnitude of \( \vec{v}_{f}(i n m / s) \) : \[ v_{f}=\left|\vec{v}_{f}\right|=\sqrt{v_{x} f^{2}+v_{y f}{ }^{2}}= \] Finalize The negative sign for the angle \( \theta \) indicates that the velocity vector is directed at an angle of \( |\theta| \) below the positive \( x \)-axis. Notice that if we calculate \( v_{j} \) from the \( x \) and \( y \)-components of \( \vec{v}_{j} \). we find that \( v_{f} \quad v_{p} \) is that consistent with our prediction? (c) Determine the \( x \) and \( y \)-coordinates of the particle at any time \( t \) and its position vector at this time. 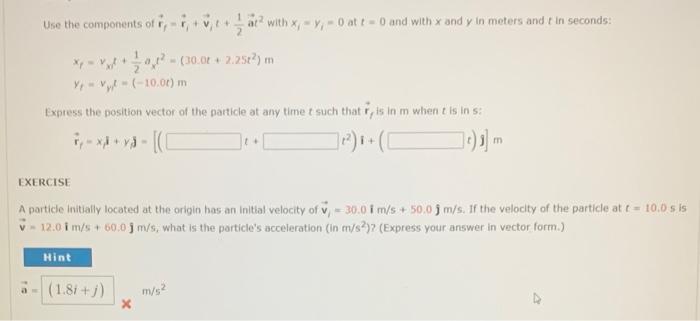SOLUTION Use the components of \( \vec{r}_{f}=\vec{r}_{i}+\vec{v}_{i} t+\frac{1}{2} \vec{a} t^{2} \) with \( x_{i}=y_{i}=0 \) at \( t=0 \) and with \( x \) and \( y \) in meters and \( t \) in seconds: \[ \begin{array}{l} x_{p}=v_{x t^{t}}+\frac{1}{2} a_{x^{2}}^{t^{2}}=\left(30.0 t+2.25 t^{2}\right) \mathrm{m} \\ y_{f}=v_{y t} t=(-10.0 t) \mathrm{m} \end{array} \] Express the position vector of the particle at any time \( t \) such that \( \vec{r}_{f} \) is in \( m \) when \( t \) is in \( s \) : \[ \vec{r}_{f}=x_{\hat{i}} \mathbf{i}+y \mathbf{j}=\left[\left(\quad t+t^{2}\right) \mathbf{i}+(\quad t) \mathbf{j}\right] \] EXERCISE A particle initially located at the origin has an initial velocity of \( \vec{v}_{i}=30.0 \mathbf{i} \mathrm{m} / \mathrm{s}+50.0 \mathrm{j} m / \mathrm{s} \). If the velocity of the particle at \( t=10.0 \mathrm{~s} \) is \( \overrightarrow{\mathbf{v}}=12.0 \mathbf{i} \mathrm{m} / \mathrm{s}+60.0 \mathbf{j} \mathrm{m} / \mathrm{s} \), what is the porticle's acceleration \( \left(\right. \) in \( \left.\mathrm{m} / \mathrm{s}^{2}\right) ? \) (Express your answer in vector form.)